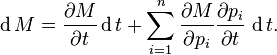Total Derivative - The Total Derivative Via Differentials

The Total Derivative Via Differentials

Differentials provide a simple way to understand the total derivative. For instance, suppose is a function of time t and n variables as in the previous section. Then, the differential of M is

This expression is often interpreted heuristically as a relation between infinitesimals. However, if the variables t and pj are interpreted as functions, and is interpreted to mean the composite of M with these functions, then the above expression makes perfect sense as an equality of differential 1-forms, and is immediate from the chain rule for the exterior derivative. The advantage of this point of view is that it takes into account arbitrary dependencies between the variables. For example, if then . In particular, if the variables pj are all functions of t, as in the previous section, then

\operatorname d M = \frac{\partial M}{\partial t} \operatorname d t + \sum_{i=1}^n \frac{\partial M}{\partial p_i}\frac{\partial p_i}{\partial t}\,\operatorname d t.

Read more about this topic:  Total Derivative

Famous quotes containing the words total and/or derivative:

    “Someone once asked me why women don’t gamble as much as men do, and I gave the common-sensical reply that we don’t have as much money. That was a true but incomplete answer. In fact, women’s total instinct for gambling is satisfied by marriage.”
    —Gloria Steinem (b. 1934)

    “Poor John Field!—I trust he does not read this, unless he will improve by it,—thinking to live by some derivative old-country mode in this primitive new country.... With his horizon all his own, yet he a poor man, born to be poor, with his inherited Irish poverty or poor life, his Adam’s grandmother and boggy ways, not to rise in this world, he nor his posterity, till their wading webbed bog-trotting feet get talaria to their heels.”
    —Henry David Thoreau (1817–1862)