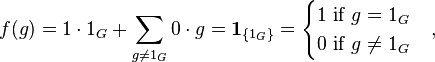Group Ring - Some Basic Properties

Some Basic Properties

Assuming that the ring R has a unit element 1, and denoting the group unit by 1G, the ring R contains a subring isomorphic to R, and its group of invertible elements contains a subgroup isomorphic to G. For considering the indicator function of {1G}, which is the vector f defined by

f(g)= 1\cdot 1_G + \sum_{g\not= 1_G}0 \cdot g= \mathbf{1}_{\{1_G\}}=\begin{cases} 1\text{ if }g = 1_G \\ 0\text{ if }g \ne 1_G \end{cases},

the set of all scalar multiples of f is a subring of R isomorphic to R. And if we map each element s of G to the indicator function of {s}, which is the vector f defined by

f(g)= 1\cdot s + \sum_{g\not= s}0 \cdot g= \mathbf{1}_{\{s\}}=\begin{cases} 1\text{ if }g = s \\ 0\text{ if }g \ne s \end{cases}

the resulting mapping is an injective group homomorphism (with respect to multiplication, not addition, in R).

If R and G are both commutative (i.e., R is commutative and G is an abelian group), R is commutative.

If H is a subgroup of G, then R is a subring of R. Similarly, if S is a subring of R, S is a subring of R.

Read more about this topic:  Group Ring

Famous quotes containing the words basic and/or properties:

    “What, then, is the basic difference between today’s computer and an intelligent being? It is that the computer can be made to see but not to perceive. What matters here is not that the computer is without consciousness but that thus far it is incapable of the spontaneous grasp of pattern—a capacity essential to perception and intelligence.”
    —Rudolf Arnheim (b. 1904)

    “The reason why men enter into society, is the preservation of their property; and the end why they choose and authorize a legislative, is, that there may be laws made, and rules set, as guards and fences to the properties of all the members of the society: to limit the power, and moderate the dominion, of every part and member of the society.”
    —John Locke (1632–1704)