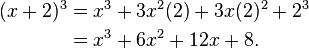Binomial Theorem - Examples

Examples

The most basic example of the binomial theorem is the formula for the square of x + y:

The binomial coefficients 1, 2, 1 appearing in this expansion correspond to the third row of Pascal's triangle. The coefficients of higher powers of x + y correspond to later rows of the triangle:

\begin{align} (x+y)^3 & = x^3 + 3x^2y + 3xy^2 + y^3, \\ (x+y)^4 & = x^4 + 4x^3y + 6x^2y^2 + 4xy^3 + y^4, \\ (x+y)^5 & = x^5 + 5x^4y + 10x^3y^2 + 10x^2y^3 + 5xy^4 + y^5, \\ (x+y)^6 & = x^6 + 6x^5y + 15x^4y^2 + 20x^3y^3 + 15x^2y^4 + 6xy^5 + y^6, \\ (x+y)^7 & = x^7 + 7x^6y + 21x^5y^2 + 35x^4y^3 + 35x^3y^4 + 21x^2y^5 + 7xy^6 + y^7. \end{align}

Notice that

  1. the powers of x go down until it reaches 0 ,starting value is n (the n in .)
  2. the powers of y go up from 0 until it reaches n (also the n in .)
  3. the nth row of the Pascal's Triangle will be the coefficients of the expanded binomial. (Note that the top is row 0.)
  4. for each line, the number of products (i.e. the sum of the coefficients) is equal to .
  5. for each line, the number of product groups is equal to .

The binomial theorem can be applied to the powers of any binomial. For example,

\begin{align} (x+2)^3 &= x^3 + 3x^2(2) + 3x(2)^2 + 2^3 \\ &= x^3 + 6x^2 + 12x + 8.\end{align}

For a binomial involving subtraction, the theorem can be applied as long as the opposite of the second term is used. This has the effect of changing the sign of every other term in the expansion:

Read more about this topic:  Binomial Theorem

Famous quotes containing the word examples:

    “It is hardly to be believed how spiritual reflections when mixed with a little physics can hold people’s attention and give them a livelier idea of God than do the often ill-applied examples of his wrath.”
    —G.C. (Georg Christoph)

    “Histories are more full of examples of the fidelity of dogs than of friends.”
    —Alexander Pope (1688–1744)

    “In the examples that I here bring in of what I have [read], heard, done or said, I have refrained from daring to alter even the smallest and most indifferent circumstances. My conscience falsifies not an iota; for my knowledge I cannot answer.”
    —Michel de Montaigne (1533–1592)