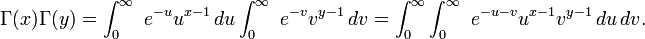Beta Function - Relationship Between Gamma Function and Beta Function

Relationship Between Gamma Function and Beta Function

To derive the integral representation of the beta function, write the product of two factorials as

\Gamma(x)\Gamma(y) = \int_0^\infty\ e^{-u} u^{x-1}\,du \int_0^\infty\ e^{-v} v^{y-1}\,dv =\int_0^\infty\int_0^\infty\ e^{-u-v} u^{x-1}v^{y-1}\,du \,dv. \!

Changing variables by putting u=zt, v=z(1-t) shows that this is

\int_{z=0}^\infty\int_{t=0}^1\ e^{-z} (zt)^{x-1}(z(1-t))^{y-1}z\,dt \,dz =\int_{z=0}^\infty \ e^{-z}z^{x+y-1} \,dz\int_{t=0}^1t^{x-1}(1-t)^{y-1}\,dt. \!

Hence

\Gamma(x)\,\Gamma(y)=\Gamma(x+y)\Beta(x,y) .

The stated identity may be seen as a particular case of the identity for the integral of a convolution. Taking

and, one has:
.

Read more about this topic:  Beta Function

Famous quotes containing the words relationship and/or function:

    “The proper aim of education is to promote significant learning. Significant learning entails development. Development means successively asking broader and deeper questions of the relationship between oneself and the world. This is as true for first graders as graduate students, for fledging artists as graying accountants.”
    —Laurent A. Daloz (20th century)

    “Science has fulfilled her function when she has ascertained and enunciated truth.”
    —Thomas Henry Huxley (1825–95)