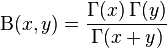Beta Function - Properties

Properties

The beta function is symmetric, meaning that

\Beta(x,y) = \Beta(y,x). \!

When x and y are positive integers, it follows trivially from the definition of the gamma function that:

\Beta(x,y)=\dfrac{(x-1)!\,(y-1)!}{(x+y-1)!} \!

It has many other forms, including:

\Beta(x,y)=\dfrac{\Gamma(x)\,\Gamma(y)}{\Gamma(x+y)} \!
\Beta(x,y) = 2\int_0^{\pi/2}(\sin\theta)^{2x-1}(\cos\theta)^{2y-1}\,d\theta, \qquad \mathrm{Re}(x)>0,\ \mathrm{Re}(y)>0 \!
\Beta(x,y) = \int_0^\infty\dfrac{t^{x-1}}{(1+t)^{x+y}}\,dt, \qquad \mathrm{Re}(x)>0,\ \mathrm{Re}(y)>0 \!
\Beta(x,y) = \sum_{n=0}^\infty \dfrac{{n-y \choose n}} {x+n}, \!
\Beta(x,y) = \frac{x+y}{x y} \prod_{n=1}^\infty \left( 1+ \dfrac{x y}{n (x+y+n)}\right)^{-1}, \!
\Beta(x,y)\cdot(t \mapsto t_+^{x+y-1}) = (t \to t_+^{x-1}) * (t \to t_+^{y-1}) \qquad x\ge 1, y\ge 1, \!
\Beta(x,y) \cdot \Beta(x+y,1-y) = \dfrac{\pi}{x \sin(\pi y)}, \!


where is a truncated power function and the star denotes convolution. The second identity shows in particular . Some of these identities, e.g. the trigonometric formula, can be applied to deriving the volume of an n-ball in Cartesian coordinates.

Euler's integral for the beta function may be converted into an integral over the Pochhammer contour C as

This Pochhammer contour integral converges for all values of α and β and so gives the analytic continuation of the beta function.

Just as the gamma function for integers describes factorials, the beta function can define a binomial coefficient after adjusting indices:

Moreover, for integer n, can be integrated to give a closed form, an interpolation function for continuous values of k:

The beta function was the first known scattering amplitude in string theory, first conjectured by Gabriele Veneziano. It also occurs in the theory of the preferential attachment process, a type of stochastic urn process.

Read more about this topic:  Beta Function

Famous quotes containing the word properties:

    “The reason why men enter into society, is the preservation of their property; and the end why they choose and authorize a legislative, is, that there may be laws made, and rules set, as guards and fences to the properties of all the members of the society: to limit the power, and moderate the dominion, of every part and member of the society.”
    —John Locke (1632–1704)

    “A drop of water has the properties of the sea, but cannot exhibit a storm. There is beauty of a concert, as well as of a flute; strength of a host, as well as of a hero.”
    —Ralph Waldo Emerson (1803–1882)