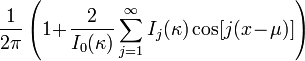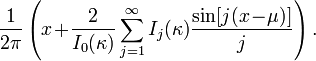Von Mises Distribution - Definition

Definition

The von Mises probability density function for the angle x is given by:

where I0(x) is the modified Bessel function of order 0.

The parameters μ and 1/κ are analogous to μ and σ2 (the mean and variance) in the normal distribution:

  • μ is a measure of location (the distribution is clustered around μ), and
  • κ is a measure of concentration (a reciprocal measure of dispersion, so 1/κ is analogous to σ2).
    • If κ is zero, the distribution is uniform, and for small κ, it is close to uniform.
    • If κ is large, the distribution becomes very concentrated about the angle μ with κ being a measure of the concentration. In fact, as κ increases, the distribution approaches a normal distribution in x with mean μ and variance 1/κ.

The probability density can be expressed as a series of Bessel functions (see Abramowitz and Stegun §9.6.34)

\frac{1}{2\pi}\left(1\!+\!\frac{2}{I_0(\kappa)} \sum_{j=1}^\infty I_j(\kappa)\cos\right)

where Ij(x) is the modified Bessel function of order j. The cumulative distribution function is not analytic and is best found by integrating the above series. The indefinite integral of the probability density is:

\frac{1}{2\pi}\left(x\!+\!\frac{2}{I_0(\kappa)} \sum_{j=1}^\infty I_j(\kappa)\frac{\sin}{j}\right).

The cumulative distribution function will be a function of the lower limit of integration x0:

Read more about this topic:  Von Mises Distribution

Famous quotes containing the word definition:

    “Although there is no universal agreement as to a definition of life, its biological manifestations are generally considered to be organization, metabolism, growth, irritability, adaptation, and reproduction.”
    —The Columbia Encyclopedia, Fifth Edition, the first sentence of the article on “life” (based on wording in the First Edition, 1935)

    “Mothers often are too easily intimidated by their children’s negative reactions...When the child cries or is unhappy, the mother reads this as meaning that she is a failure. This is why it is so important for a mother to know...that the process of growing up involves by definition things that her child is not going to like. Her job is not to create a bed of roses, but to help him learn how to pick his way through the thorns.”
    —Elaine Heffner (20th century)

    “Scientific method is the way to truth, but it affords, even in
    principle, no unique definition of truth. Any so-called pragmatic
    definition of truth is doomed to failure equally.”
    —Willard Van Orman Quine (b. 1908)