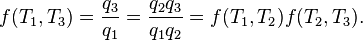Thermodynamic Temperature - Definition of Thermodynamic Temperature

Definition of Thermodynamic Temperature

Strictly speaking, the temperature of a system is well-defined only if its particles (atoms, molecules, electrons, photons) are at equilibrium, so that their energies obey a Boltzmann distribution (or its quantum mechanical counterpart). There are many possible scales of temperature, derived from a variety of observations of physical phenomena. The thermodynamic temperature can be shown to have special properties, and in particular can be seen to be uniquely defined (up to some constant multiplicative factor) by considering the efficiency of idealized heat engines. Thus the ratio T2/T1 of two temperaturesT1 andT2 is the same in all absolute scales.

Loosely stated, temperature controls the flow of heat between two systems, and the universe as a whole, as with any natural system, tends to progress so as to maximize entropy. This suggests that there should be a relationship between temperature and entropy. To elucidate this, consider first the relationship between heat, work and temperature. One way to study this is to analyze a heat engine, which is a device for converting heat into mechanical work, such as the Carnot heat engine. Such a heat engine functions by using a temperature gradient between a high temperatureTH and a low temperature TC to generate work, and the work done (per cycle, say) by the heat engine is equal to the difference between the heat energy qH put into the system at the high temperature and the heat qC ejected at the low temperature (in that cycle). The efficiency of the engine is the work divided by the heat put into the system or

where wcy is the work done per cycle. Thus the efficiency depends only on qC/qH.

Carnot's theorem states that all reversible engines operating between the same heat reservoirs are equally efficient. Thus, any reversible heat engine operating between temperatures T1 and T2 must have the same efficiency, that is to say, the efficiency is the function of only temperatures

In addition, a reversible heat engine operating between temperatures T1 and T3 must have the same efficiency as one consisting of two cycles, one between T1 and another (intermediate) temperature T2, and the second between T2 andT3. A quick way to see this is that should this not be the case, then energy (in the form of Q) will be wasted or gained, resulting in different overall efficiencies every time a cycle is split into component cycles; clearly a cycle can be composed of any number of smaller cycles.

With this understanding of Q1, Q2 and Q3, we note also that mathematically,

f(T_1,T_3) = \frac{q_3}{q_1} = \frac{q_2 q_3} {q_1 q_2} = f(T_1,T_2)f(T_2,T_3).

But the first function is NOT a function of T2, therefore the product of the final two functions MUST result in the removal of T2 as a variable. The only way is therefore to define the function f as follows:

and

so that

i.e. The ratio of heat exchanged is a function of the respective temperatures at which they occur. We can choose any monotonic function for our ; it is a matter of convenience and convention that we choose . Choosing then one fixed reference temperature (i.e. triple point of water), we establish the thermodynamic temperature scale.

It is to be noted that such a definition coincides with that of the ideal gas derivation; also it is this definition of the thermodynamic temperature that enables us to represent the Carnot efficiency in terms of TH and TC, and hence derive that the (complete) Carnot cycle is isentropic:

Substituting this back into our first formula for efficiency yields a relationship in terms of temperature:

Notice that for TC=0 the efficiency is 100% and that efficiency becomes greater than 100% for TC<0, which cases are unrealistic. Subtracting the right hand side of Equation 4 from the middle portion and rearranging gives

where the negative sign indicates heat ejected from the system. The generalization of this equation is Clausius theorem, which suggests the existence of a state function S (i.e., a function which depends only on the state of the system, not on how it reached that state) defined (up to an additive constant) by

where the subscript indicates heat transfer in a reversible process. The function S corresponds to the entropy of the system, mentioned previously, and the change of S around any cycle is zero (as is necessary for any state function). Equation 5 can be rearranged to get an alternative definition for temperature in terms of entropy and heat (to avoid logic loop, we should first define entropy through statistical mechanics):

For a system in which the entropy S is a function S(E) of its energy E, the thermodynamic temperature T is therefore given by

so that the reciprocal of the thermodynamic temperature is the rate of increase of entropy with energy.

Read more about this topic:  Thermodynamic Temperature

Famous quotes containing the words definition of, definition and/or temperature:

    “It is very hard to give a just definition of love. The most we can say of it is this: that in the soul, it is a desire to rule; in the spirit, it is a sympathy; and in the body, it is but a hidden and subtle desire to possess—after many mysteries—what one loves.”
    —François, Duc De La Rochefoucauld (1613–1680)

    “... if, as women, we accept a philosophy of history that asserts that women are by definition assimilated into the male universal, that we can understand our past through a male lens—if we are unaware that women even have a history—we live our lives similarly unanchored, drifting in response to a veering wind of myth and bias.”
    —Adrienne Rich (b. 1929)

    “The siren south is well enough, but New York, at the beginning of March, is a hoyden we would not care to miss—a drafty wench, her temperature up and down, full of bold promises and dust in the eye.”
    —E.B. (Elwyn Brooks)