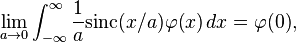Relationship To The Dirac Delta Distribution
The normalized sinc function can be used as a nascent delta function, meaning that the following weak limit holds:
This is not an ordinary limit, since the left side does not converge. Rather, it means that
for any smooth function with compact support.
In the above expression, as a approaches zero, the number of oscillations per unit length of the sinc function approaches infinity. Nevertheless, the expression always oscillates inside an envelope of ±1/(π a x), and approaches zero for any nonzero value of x. This complicates the informal picture of δ(x) as being zero for all x except at the point x = 0 and illustrates the problem of thinking of the delta function as a function rather than as a distribution. A similar situation is found in the Gibbs phenomenon.
Read more about this topic: Sinc Function
Famous quotes containing the words relationship to the, relationship to, relationship and/or distribution:
“Film music should have the same relationship to the film drama that somebody’s piano playing in my living room has to the book I am reading.”
—Igor Stravinsky (1882–1971)
“Sometimes in our relationship to another human being the proper balance of friendship is restored when we put a few grains of impropriety onto our own side of the scale.”
—Friedrich Nietzsche (1844–1900)
“It would be a fallacy to deduce that the slow writer necessarily comes up with superior work. There seems to be scant relationship between prolificness and quality.”
—Fannie Hurst (1889–1968)
“In this distribution of functions, the scholar is the delegated intellect. In the right state, he is, Man Thinking. In the degenerate state, when the victim of society, he tends to become a mere thinker, or, still worse, the parrot of other men’s thinking.”
—Ralph Waldo Emerson (1803–1882)