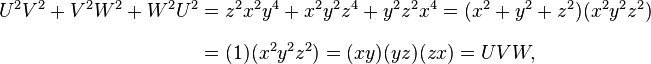Roman Surface - Derivation of Implicit Formula

Derivation of Implicit Formula

For simplicity we consider only the case r = 1. Given the sphere defined by the points (x, y, z) such that

we apply to these points the transformation T defined by

say.

But then we have

and so

as desired.

Conversely, suppose we are given (U, V, W) satisfying

(*)

We prove that there exists (x,y,z) such that

(**)

for which

with one exception: In case 3.b. below, we show this cannot be proved.

1. In the case where none of U, V, W is 0, we can set

(Note that (*) guarantees that either all three of U, V, W are positive, or else exactly two are negative. So these square roots are of positive numbers.)

It is easy to use (*) to confirm that (**) holds for x, y, z defined this way.

2. Suppose that W is 0. From (*) this implies

and hence at least one of U, V must be 0 also. This shows that is it impossible for exactly one of U, V, W to be 0.

3. Suppose that exactly two of U, V, W are 0. Without loss of generality we assume

(***)

It follows that

(since

implies that

and hence

contradicting (***).)

a. In the subcase where

if we determine x and y by

and

this ensures that (*) holds. It is easy to verify that

and hence choosing the signs of x and y appropriately will guarantee

Since also

this shows that this subcase leads to the desired converse.

b. In this remaining subcase of the case 3., we have

Since

it is easy to check that

and thus in this case, where

there is no (x, y, z) satisfying

Hence the solutions (U, 0, 0) of the equation (*) with

and likewise, (0, V, 0) with

and (0, 0, W) with

(each of which is a noncompact portion of a coordinate axis, in two pieces) do not correspond to any point on the Roman surface.

4. If (U, V, W) is the point (0, 0, 0), then if any two of x, y, z are zero and the third one has absolute value 1, clearly

as desired.

This covers all possible cases.