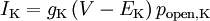Quantitative Models of The Action Potential - Hodgkin-Huxley Model

Hodgkin-Huxley Model

In 1952 Alan Lloyd Hodgkin and Andrew Huxley developed a set of equations to fit their experimental voltage-clamp data on the axonal membrane. The model assumes that the membrane capacitance C is constant; thus, the transmembrane voltage V changes with the total transmembrane current I_tot according to the equation

where I_Na, I_K, and I_L are currents conveyed through the local sodium channels, potassium channels, and "leakage" channels (a catch-all), respectively. The initial term I_ext represents the current arriving from external sources, such as excitatory postsynaptic potentials from the dendrites or a scientist's electrode.

The model further assumes that a given ion channel is either fully open or closed; if closed, its conductance is zero, whereas if open, its conductance is some constant value g. Hence, the net current through an ion channel depends on two variables: the probability p_open of the channel being open, and the difference in voltage from that ion's equilibrium voltage, V − V_eq. For example, the current through the potassium channel may be written as

which is equivalent to Ohm's law. By definition, no net current flows (I_K = 0) when the transmembrane voltage equals the equilibrium voltage of that ion (when V = E_K).

To fit their data accurately, Hodgkin and Huxley assumed that each type of ion channel had multiple "gates", so that the channel was open only if all the gates were open and closed otherwise. They also assumed that the probability of a gate being open was independent of the other gates being open; this assumption was later validated for the inactivation gate. Hodgkin and Huxley modeled the voltage-sensitive potassium channel as having four gates; letting p_n denote the probability of a single such gate being open, the probability of the whole channel being open is the product of four such probabilities, i.e., p_{open, K} = n4. Similarly, the probability of the voltage-sensitive sodium channel was modeled to have three similar gates of probability m and a fourth gate, associated with inactivation, of probability h; thus, p_{open, Na} = m3h. The probabilities for each gate are assumed to obey first-order kinetics

where both the equilibrium value m_eq and the relaxation time constant τ_m depend on the instantaneous voltage V across the membrane. If V changes on a time-scale more slowly than τ_m, the m probability will always roughly equal its equilibrium value m_eq; however, if V changes more quickly, then m will lag behind m_eq. By fitting their voltage-clamp data, Hodgkin and Huxley were able to model how these equilibrium values and time constants varied with temperature and transmembrane voltage. The formulae are complex and depend exponentially on the voltage and temperature. For example, the time constant for sodium-channel activation probability h varies as 3(θ−6.3)/10 with the Celsius temperature θ, and with voltage V as

In summary, the Hodgkin-Huxley equations are complex, non-linear ordinary differential equations in four independent variables: the transmembrane voltage V, and the probabilities m, h and n. No general solution of these equations has been discovered. A less ambitious but generally applicable method for studying such non-linear dynamical systems is to consider their behavior in the vicinity of a fixed point. This analysis shows that the Hodgkin-Huxley system undergoes a transition from stable quiescence to bursting oscillations as the stimulating current I_ext is gradually increased; remarkably, the axon becomes stably quiescent again as the stimulating current is increased further still. A more general study of the types of qualitative behavior of axons predicted by the Hodgkin-Huxley equations has also been carried out.