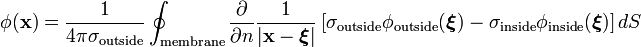Quantitative Models of The Action Potential - Extracellular Potentials and Currents

Extracellular Potentials and Currents

Whereas the above models simulate the transmembrane voltage and current at a single patch of membrane, other mathematical models pertain to the voltages and currents in the ionic solution surrounding the neuron. Such models are helpful in interpreting data from extracellular electrodes, which were common prior to the invention of the glass pipette electrode that allowed intracellular recording. The extracellular medium may be modeled as a normal isotropic ionic solution; in such solutions, the current follows the electric field lines, according to the continuum form of Ohm's Law

where j and E are vectors representing the current density and electric field, respectively, and where σ is the conductivity. Thus, j can be found from E, which in turn may be found using Maxwell's equations. Maxwell's equations can be reduced to a relatively simple problem of electrostatics, since the ionic concentrations change too slowly (compared to the speed of light) for magnetic effects to be important. The electric potential φ(x) at any extracellular point x can be solved using Green's identities

where the integration is over the complete surface of the membrane; is a position on the membrane, σ_inside and φ_inside are the conductivity and potential just within the membrane, and σ_outside and φ_outside the corresponding values just outside the membrane. Thus, given these σ and φ values on the membrane, the extracellular potential φ(x) can be calculated for any position x; in turn, the electric field E and current density j can be calculated from this potential field.