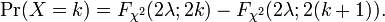Poisson Distribution - Related Distributions

Related Distributions

• If and are independent, then the difference follows a Skellam distribution.
• If and are independent, then the distribution of conditional on is a binomial distribution. Specifically, given, . More generally, if X₁, X₂,..., X_n are independent Poisson random variables with parameters λ₁, λ₂,..., λ_n then

given . In fact, .

• If and the distribution of, conditional on X = k, is a binomial distribution, then the distribution of Y follows a Poisson distribution . In fact, if, conditional on X = k, follows a multinomial distribution, then each follows an independent Poisson distribution .
• The Poisson distribution can be derived as a limiting case to the binomial distribution as the number of trials goes to infinity and the expected number of successes remains fixed — see law of rare events below. Therefore it can be used as an approximation of the binomial distribution if n is sufficiently large and p is sufficiently small. There is a rule of thumb stating that the Poisson distribution is a good approximation of the binomial distribution if n is at least 20 and p is smaller than or equal to 0.05, and an excellent approximation if n ≥ 100 and np ≤ 10.

• The Poisson distribution is a special case of generalized stuttering Poisson distribution (or stuttering Poisson distribution) with only a parameter. Stuttering Poisson distribution can be deduced from the limiting distribution of multinomial distribution.
• For sufficiently large values of λ, (say λ>1000), the normal distribution with mean λ and variance λ (standard deviation ), is an excellent approximation to the Poisson distribution. If λ is greater than about 10, then the normal distribution is a good approximation if an appropriate continuity correction is performed, i.e., P(X ≤ x), where (lower-case) x is a non-negative integer, is replaced by P(X ≤ x + 0.5).

• Variance-stabilizing transformation: When a variable is Poisson distributed, its square root is approximately normally distributed with expected value of about and variance of about 1/4. Under this transformation, the convergence to normality (as λ increases) is far faster than the untransformed variable. Other, slightly more complicated, variance stabilizing transformations are available, one of which is Anscombe transform. See Data transformation (statistics) for more general uses of transformations.
• If for every t > 0 the number of arrivals in the time interval follows the Poisson distribution with mean λ t, then the sequence of inter-arrival times are independent and identically distributed exponential random variables having mean 1 / λ.
• The cumulative distribution functions of the Poisson and chi-squared distributions are related in the following ways:

and