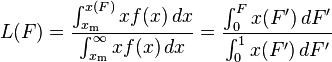Pareto Distribution - Lorenz Curve and Gini Coefficient

Lorenz Curve and Gini Coefficient

The Lorenz curve is often used to characterize income and wealth distributions. For any distribution, the Lorenz curve L(F) is written in terms of the PDF ƒ or the CDF F as

$L(F)=\frac{\int_{x_\mathrm{m}}^{x(F)} xf(x)\,dx}{\int_{x_\mathrm{m}}^\infty xf(x)\,dx} =\frac{\int_0^F x(F')\,dF'}{\int_0^1 x(F')\,dF'}$

where x(F) is the inverse of the CDF. For the Pareto distribution,

and the Lorenz curve is calculated to be

where α must be greater than or equal to unity, since the denominator in the expression for L(F) is just the mean value of x. Examples of the Lorenz curve for a number of Pareto distributions are shown in the graph on the right.

The Gini coefficient is a measure of the deviation of the Lorenz curve from the equidistribution line which is a line connecting and, which is shown in black (α = ∞) in the Lorenz plot on the right. Specifically, the Gini coefficient is twice the area between the Lorenz curve and the equidistribution line. The Gini coefficient for the Pareto distribution is then calculated to be

(see Aaberge 2005).

Read more about this topic: Pareto Distribution

Famous quotes containing the word curve:

“The years-heired feature that can
In curve and voice and eye
Despise the human span
Of durance—that is I;
The eternal thing in man,
That heeds no call to die.”
—Thomas Hardy (1840–1928)