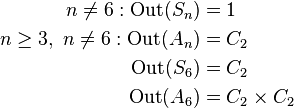Outer Automorphism Group - The Outer Automorphisms of The Symmetric and Alternating Groups

The Outer Automorphisms of The Symmetric and Alternating Groups

For more details on this topic, see Automorphisms of the symmetric and alternating groups.

The outer automorphism group of a finite simple group in some infinite family of finite simple groups can almost always be given by a uniform formula that works for all elements of the family. There is just one exception to this: the alternating group A6 has outer automorphism group of order 4, rather than 2 as do the other simple alternating groups (given by conjugation by an odd permutation). Equivalently the symmetric group S6 is the only symmetric group with a non-trivial outer automorphism group.

\begin{align} n\neq 6: \mathrm{Out}(S_n) & = 1 \\ n\geq 3,\ n\neq 6: \mathrm{Out}(A_n) & = C_2 \\ \mathrm{Out}(S_6) & = C_2 \\ \mathrm{Out}(A_6) & = C_2 \times C_2 \end{align}

Read more about this topic:  Outer Automorphism Group

Famous quotes containing the words outer and/or groups:

    “I know, it must have been my imagination, but it makes me realize how desperately alone the Earth is. Hanging in space like a speck of food floating in the ocean. Sooner or later to be swallowed up by some creature floating by.... Time will tell, Dr. Mason. We can only wait and wonder. Wonder how, wonder when.”
    —Tom Graeff. Young astronomer, Teenagers from Outer Space, after just seeing the invading spaceship through his telescope, and dismissing it (1959)

    “As in political revolutions, so in paradigm choice—there is no standard higher than the assent of the relevant community. To discover how scientific revolutions are effected, we shall therefore have to examine not only the impact of nature and of logic, but also the techniques of persuasive argumentation effective within the quite special groups that constitute the community of scientists.”
    —Thomas S. Kuhn (b. 1922)