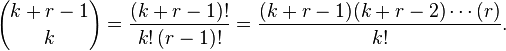Negative Binomial Distribution - Definition

Definition

Suppose there is a sequence of independent Bernoulli trials, each trial having two potential outcomes called “success” and “failure”. In each trial the probability of success is p and of failure is (1 − p). We are observing this sequence until a predefined number r of failures has occurred. Then the random number of successes we have seen, X, will have the negative binomial (or Pascal) distribution:

X\ \sim\ \text{NB}(r,\, p)

When applied to real-world problems, outcomes of success and failure may or may not be outcomes we ordinarily view as good and bad, respectively. Suppose we used the negative binomial distribution to model the number of days a certain machine works before it breaks down. In this case “success” would be the result on a day when the machine worked properly, whereas a breakdown would be a “failure”. If we used the negative binomial distribution to model the number of goal attempts a sportsman makes before scoring a goal, though, then each unsuccessful attempt would be a “success”, and scoring a goal would be “failure”. If we are tossing a coin, then the negative binomial distribution can give the number of heads (“success”) we are likely to encounter before we encounter a certain number of tails (“failure”).

The probability mass function of the negative binomial distribution is

f(k) \equiv \Pr(X = k) = {k+r-1 \choose k} (1-p)^r p^k \quad\text{for }k = 0, 1, 2, \dots

Here the quantity in parentheses is the binomial coefficient, and is equal to

{k+r-1 \choose k} = \frac{(k+r-1)!}{k!\,(r-1)!} = \frac{(k+r-1)(k+r-2)\cdots(r)}{k!}.

This quantity can alternatively be written in the following manner, explaining the name “negative binomial”:

\frac{(k+r-1)\cdots(r)}{k!} = (-1)^k \frac{(-r)(-r-1)(-r-2)\cdots(-r-k+1)}{k!} = (-1)^k{-r \choose k}. \qquad (*)

To understand the above definition of the probability mass function, note that the probability for every specific sequence of k successes and r failures is (1 − p)rpk, because the outcomes of the k + r trials are supposed to happen independently. Since the rth failure comes last, it remains to choose the k trials with successes out of the remaining k + r − 1 trials. The above binomial coefficient, due to its combinatorial interpretation, gives precisely the number of all these sequences of length k + r − 1.

Read more about this topic:  Negative Binomial Distribution

Famous quotes containing the word definition:

    “One definition of man is “an intelligence served by organs.””
    —Ralph Waldo Emerson (1803–1882)

    “Perhaps the best definition of progress would be the continuing efforts of men and women to narrow the gap between the convenience of the powers that be and the unwritten charter.”
    —Nadine Gordimer (b. 1923)

    “It is very hard to give a just definition of love. The most we can say of it is this: that in the soul, it is a desire to rule; in the spirit, it is a sympathy; and in the body, it is but a hidden and subtle desire to possess—after many mysteries—what one loves.”
    —François, Duc De La Rochefoucauld (1613–1680)