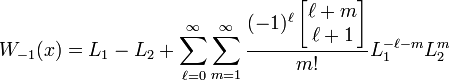Lambert W Function - Asymptotic Expansions

Asymptotic Expansions

The Taylor series of around 0 can be found using the Lagrange inversion theorem and is given by

W_0 (x) = \sum_{n=1}^\infty \frac{(-n)^{n-1}}{n!}\ x^n = x - x^2 + \frac{3}{2}x^3 - \frac{8}{3}x^4 + \frac{125}{24}x^5 - \cdots

The radius of convergence is 1/e, as may be seen by the ratio test. The function defined by this series can be extended to a holomorphic function defined on all complex numbers with a branch cut along the interval (−∞, −1/e]; this holomorphic function defines the principal branch of the Lambert W function.

An asymptotic expansion for the other real branch, defined in the interval (−∞, −1/e], is

W_{-1} (x) = L_1 - L_2 + \frac{L_2}{L_1} + \frac{L_2 (-2 + L_2)}{2 L_1^2} + \frac{ L_2 (6 - 9 L_2 + 2 L_2^2) }{6 L_1^3} + \frac{L_2 (-12+36L_2 - 22 L_2^2 + 3 L_2^3)}{12 L_1^4} + \cdots
W_{-1} (x) = L_1-L_2+\sum_{\ell=0}^{\infty}\sum_{m=1}^{\infty}\frac{(-1)^{\ell}\left }{m!} L_1^{-\ell-m} L_2^{m}

where and and is a non-negative Stirling number of the first kind.

Read more about this topic:  Lambert W Function