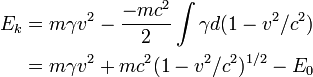Kinetic Energy - Relativistic Kinetic Energy of Rigid Bodies

Relativistic Kinetic Energy of Rigid Bodies

See also: Mass in special relativity and Tests of relativistic energy and momentum

In special relativity, we must change the expression for linear momentum.

Using m for rest mass, v and v for the object's velocity and speed respectively, and c for the speed of light in vacuum, we assume for linear momentum that, where .

Integrating by parts gives

Remembering that, we get:

\begin{align} E_k &= m \gamma v^2 - \frac{- m c^2}{2} \int \gamma d (1 - v^2/c^2) \\ &= m \gamma v^2 + m c^2 (1 - v^2/c^2)^{1/2} - E_0 \end{align}

where E0 serves as an integration constant. Thus:

\begin{align} E_k &= m \gamma (v^2 + c^2 (1 - v^2/c^2)) - E_0 \\ &= m \gamma (v^2 + c^2 - v^2) - E_0 \\ &= m \gamma c^2 - E_0 \end{align}

The constant of integration E0 is found by observing that, when and, giving

and giving the usual formula:

If a body's speed is a significant fraction of the speed of light, it is necessary to use relativistic mechanics (the theory of relativity as developed by Albert Einstein) to calculate its kinetic energy.

For a relativistic object the momentum p is equal to:

.

Thus the work expended accelerating an object from rest to a relativistic speed is:

.

The equation shows that the energy of an object approaches infinity as the velocity v approaches the speed of light c, thus it is impossible to accelerate an object across this boundary.

The mathematical by-product of this calculation is the mass-energy equivalence formula—the body at rest must have energy content equal to:

At a low speed (v<

,

So, the total energy E can be partitioned into the energy of the rest mass plus the traditional Newtonian kinetic energy at low speeds.

When objects move at a speed much slower than light (e.g. in everyday phenomena on Earth), the first two terms of the series predominate. The next term in the approximation is small for low speeds, and can be found by extending the expansion into a Taylor series by one more term:

.

For example, for a speed of 10 km/s (22,000 mph) the correction to the Newtonian kinetic energy is 0.0417 J/kg (on a Newtonian kinetic energy of 50 MJ/kg) and for a speed of 100 km/s it is 417 J/kg (on a Newtonian kinetic energy of 5 GJ/kg), etc.

For higher speeds, the formula for the relativistic kinetic energy is derived by simply subtracting the rest mass energy from the total energy:

.

The relation between kinetic energy and momentum is more complicated in this case, and is given by the equation:

.

This can also be expanded as a Taylor series, the first term of which is the simple expression from Newtonian mechanics.

What this suggests is that the formulas for energy and momentum are not special and axiomatic, but rather concepts which emerge from the equation of mass with energy and the principles of relativity.

Read more about this topic:  Kinetic Energy

Famous quotes containing the words kinetic, energy, rigid and/or bodies:

    “The poem has a social effect of some kind whether or not the poet wills it to have. It has kinetic force, it sets in motion ... [ellipsis in source] elements in the reader that would otherwise be stagnant.”
    —Denise Levertov (b. 1923)

    “Perhaps catastrophe is the natural human environment, and even though we spend a good deal of energy trying to get away from it, we are programmed for survival amid catastrophe.”
    —Germaine Greer (b. 1939)

    “We are beginning a new era in our government. I cannot too strongly urge the necessity of a rigid economy and an inflexible determination not to enlarge the income beyond the real necessities of the government.”
    —Andrew Jackson (1767–1845)

    “A mob is a society of bodies voluntarily bereaving themselves of reason, and traversing its work. The mob is man voluntarily descending to the nature of the beast.”
    —Ralph Waldo Emerson (1803–1882)