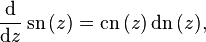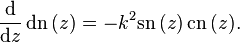Jacobi Elliptic Functions As Solutions of Nonlinear Ordinary Differential Equations
The derivatives of the three basic Jacobi elliptic functions are:
With the addition theorems above and for a given k with 0 < k < 1 they therefore are solutions to the following nonlinear ordinary differential equations:
- solves the differential equations
-
- and
- solves the differential equations
-
- and
- solves the differential equations
-
- and
Read more about this topic: Jacobi Elliptic Functions
Famous quotes containing the words jacobi, functions, solutions, ordinary and/or differential:
“... spinsterhood [is considered to be] an abnormality of small proportions and small consequence, something like an extra finger or two on the body, presumably of temporary duration, and never of any social significance.”
—Mary Putnam Jacobi (1842–1906)
“Nobody is so constituted as to be able to live everywhere and anywhere; and he who has great duties to perform, which lay claim to all his strength, has, in this respect, a very limited choice. The influence of climate upon the bodily functions ... extends so far, that a blunder in the choice of locality and climate is able not only to alienate a man from his actual duty, but also to withhold it from him altogether, so that he never even comes face to face with it.”
—Friedrich Nietzsche (1844–1900)
“Every man is in a state of conflict, owing to his attempt to reconcile himself and his relationship with life to his conception of harmony. This conflict makes his soul a battlefield, where the forces that wish this reconciliation fight those that do not and reject the alternative solutions they offer. Works of art are attempts to fight out this conflict in the imaginative world.”
—Rebecca West (1892–1983)
“It appears that ordinary men take wives because possession is not possible without marriage, and that ordinary women accept husbands because marriage is not possible without possession; with totally differing aims the method is the same on both sides.”
—Thomas Hardy (1840–1928)
“But how is one to make a scientist understand that there is something unalterably deranged about differential calculus, quantum theory, or the obscene and so inanely liturgical ordeals of the precession of the equinoxes.”
—Antonin Artaud (1896–1948)