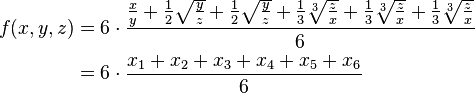Inequality of Arithmetic and Geometric Means - Example Application

Example Application

Consider the function

for all positive real numbers x, y and z. Suppose we wish to find the minimal value of this function. First we rewrite it a bit:

\begin{align} f(x,y,z) &= 6 \cdot \frac{ \frac{x}{y} + \frac{1}{2} \sqrt{\frac{y}{z}} + \frac{1}{2} \sqrt{\frac{y}{z}} + \frac{1}{3} \sqrt{\frac{z}{x}} + \frac{1}{3} \sqrt{\frac{z}{x}} + \frac{1}{3} \sqrt{\frac{z}{x}} }{6}\\ &=6\cdot\frac{x_1+x_2+x_3+x_4+x_5+x_6}{6} \end{align}

with

Applying the AM–GM inequality for n = 6, we get

\begin{align} f(x,y,z) &\ge 6 \cdot \sqrt{ \frac{x}{y} \cdot \frac{1}{2} \sqrt{\frac{y}{z}} \cdot \frac{1}{2} \sqrt{\frac{y}{z}} \cdot \frac{1}{3} \sqrt{\frac{z}{x}} \cdot \frac{1}{3} \sqrt{\frac{z}{x}} \cdot \frac{1}{3} \sqrt{\frac{z}{x}} }\\ &= 6 \cdot \sqrt{ \frac{1}{2 \cdot 2 \cdot 3 \cdot 3 \cdot 3} \frac{x}{y} \frac{y}{z} \frac{z}{x} }\\ &= 2^{2/3} \cdot 3^{1/2}. \end{align}

Further, we know that the two sides are equal exactly when all the terms of the mean are equal:

All the points (x,y,z) satisfying these conditions lie on a half-line starting at the origin and are given by

Read more about this topic:  Inequality Of Arithmetic And Geometric Means

Famous quotes containing the word application:

    “The best political economy is the care and culture of men; for, in these crises, all are ruined except such as are proper individuals, capable of thought, and of new choice and the application of their talent to new labor.”
    —Ralph Waldo Emerson (1803–1882)

    “Great abilites are not requisite for an Historian; for in historical composition, all the greatest powers of the human mind are quiescent. He has facts ready to his hand; so there is no exercise of invention. Imagination is not required in any degree; only about as much as is used in the lowest kinds of poetry. Some penetration, accuracy, and colouring, will fit a man for the task, if he can give the application which is necessary.”
    —Samuel Johnson (1709–1784)