Deriving Hamilton's Equations
Hamilton's equations can be derived by looking at how the total differential of the Lagrangian depends on time, generalized positions and generalized velocities
Now the generalized momenta were defined as and Lagrange's equations tell us that
We can rearrange this to get
and substitute the result into the total differential of the Lagrangian
We can rewrite this as
and rearrange again to get
The term on the left-hand side is just the Hamiltonian that we have defined before, so we find that
where the second equality holds because of the definition of the total differential of in terms of its partial derivatives. Associating terms from both sides of the equation above yields Hamilton's equations
Read more about this topic: Hamiltonian Mechanics
Famous quotes containing the words deriving and/or hamilton:
“Today’s pressures on middle-class children to grow up fast begin in early childhood. Chief among them is the pressure for early intellectual attainment, deriving from a changed perception of precocity. Several decades ago precocity was looked upon with great suspicion. The child prodigy, it was thought, turned out to be a neurotic adult; thus the phrase “early ripe, early rot!””
—David Elkind (20th century)
“A people’s literature is the great textbook for real knowledge of them. The writings of the day show the quality of the people as no historical reconstruction can.”
—Edith Hamilton (1867–1963)
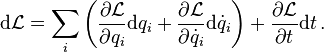





![\mathrm{d} \mathcal{H} = \sum_i \left - \frac{\partial \mathcal{L}}{\partial t}\mathrm{d}t = \sum_i \left [ \frac{\partial \mathcal{H}}{\partial q_i} \mathrm{d} q_i +
\frac{\partial \mathcal{H}}{\partial p_i} \mathrm{d} p_i \right ] + \frac{\partial \mathcal{H}}{\partial t}\mathrm{d}t
\,](http://upload.wikimedia.org/math/8/c/d/8cddf34af49f16630ea85ec57736cff9.png)