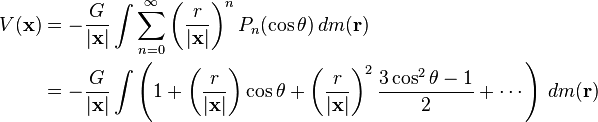Gravitational Potential - Multipole Expansion

Multipole Expansion

The potential at a point x is given by

The potential can be expanded in a series of Legendre polynomials. Represent the points x and r as position vectors relative to the center of mass. The denominator in the integral is expressed as the square root of the square to give

\begin{align} V(\mathbf{x}) &= - \int_{\mathbb{R}^3} \frac{G}{ \sqrt{|\mathbf{x}|^2 -2 \mathbf{x} \cdot \mathbf{r} + |\mathbf{r}|^2}}\,dm(\mathbf{r})\\ {}&=- \frac{1}{|\mathbf{x}|}\int_{\mathbb{R}^3} G \, \left/ \, \sqrt{1 -2 \frac{r}{|\mathbf{x}|} \cos \theta + \left( \frac{r}{|\mathbf{x}|} \right)^2}\right.\,dm(\mathbf{r}) \end{align}

where in the last integral, r = |r| and θ is the angle between x and r.

The integrand can be expanded as a Taylor series in Z = r/|x|, by explicit calculation of the coefficients. A less laborious way of achieving the same result is by using the generalized binomial theorem. The resulting series is the generating function for the Legendre polynomials:

valid for |X| ≤ 1 and |Z| < 1. The coefficients Pn are the Legendre polynomials of degree n. Therefore, the Taylor coefficients of the integrand are given by the Legendre polynomials in X = cos θ. So the potential can be expanded in a series that is convergent for positions x such that r < |x| for all mass elements of the system (i.e., outside a sphere, centered at the center of mass, that encloses the system):

\begin{align} V(\mathbf{x}) &= - \frac{G}{|\mathbf{x}|} \int \sum_{n=0}^\infty \left(\frac{r}{|\mathbf{x}|} \right)^n P_n(\cos \theta) \, dm(\mathbf{r})\\ {}&= - \frac{G}{|\mathbf{x}|} \int \left(1 + \left(\frac{r}{|\mathbf{x}|}\right) \cos \theta + \left(\frac{r}{|\mathbf{x}|}\right)^2\frac {3 \cos^2 \theta - 1}{2} + \cdots\right)\,dm(\mathbf{r}) \end{align}

The integral is the component of the center of mass in the x direction; this vanishes because the vector x emanates from the center of mass. So, bringing the integral under the sign of the summation gives

This shows that elongation of the body causes a lower potential in the direction of elongation, and a higher potential in perpendicular directions, compared to the potential due to a spherical mass, if we compare cases with the same distance to the center of mass. (If we compare cases with the same distance to the surface the opposite is true.)

Read more about this topic:  Gravitational Potential

Famous quotes containing the word expansion:

    “Artistic genius is an expansion of monkey imitativeness.”
    —W. Winwood Reade (1838–1875)