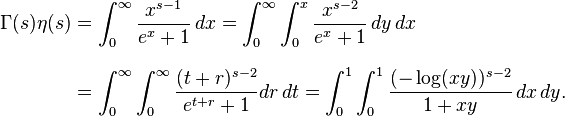Dirichlet Eta Function - Integral Representations

Integral Representations

A number of integral formulas involving the eta function can be listed. The first one follows from a change of variable of the integral representation of the Gamma function (Abel, 1823), giving a Mellin transform which can be expressed in different ways as a double integral (Sondow, 2005). This is valid for

\begin{align} \Gamma(s)\eta(s) & = \int_0^\infty \frac{x^{s-1}}{e^x+1} \, dx = \int_0^\infty \int_0^x \frac{x^{s-2}}{e^x+1} \, dy \, dx \\ & =\int_0^\infty\int_0^\infty \frac{(t+r)^{s-2}}{e^{t+r}+1}{dr} \, dt =\int_0^1\int_0^1 \frac{(-\log(x y))^{s-2}}{1 + x y} \, dx \, dy. \end{align}

The Cauchy–Schlömilch transformation (Amdeberhan, Moll et al., 2010) can be used to prove this other representation, valid for . Integration by parts of the first integral above in this section yields another derivation.

2^{1-s}\,\Gamma(s+1)\,\eta(s) = 2 \int_0^\infty \frac{x^{2s+1}}{\cosh^2(x^2)} \, dx = \int_0^\infty \frac{t^s}{\cosh^2(t)} \, dt.

The next formula, due to Lindelöf (1905), is valid over the whole complex plane, when the principal value is taken for the logarithm implicit in the exponential.

\eta(s) = \int_{-\infty}^\infty \frac{(1/2 + i t)^{-s}}{e^{\pi t}+e^{-\pi t}} \, dt.

This corresponds to a Jensen (1895) formula for the entire function, valid over the whole complex plane and also proven by Lindelöf.

(s-1)\zeta(s) = \int_{-\infty}^\infty \frac{(1/2 + i t)^{1-s}}{(e^{\pi t}+e^{-\pi t})^2} \, dt.

"This formula, remarquable by its simplicity, can be proven easily with the help Cauchy's theorem, so important for the summation of series" wrote Jensen (1895). Similarly by converting the integration paths to contour integrals one can obtain other formulas for the eta function, such as this generalisation (Milgram, 2012, formula 2.9) stated to be valid for and all :

\eta(s) = \frac{1}{2} \int_{-\infty}^\infty \frac{(c + i t)^{-s}}{\sin{(\pi(c+i t))}} \, dt.

The zeros on the negative real axis are factored out cleanly by making (Milgram, 2012, formula 3.9) stated to be valid for :

\eta(s) = - \sin(s\pi/2) \int_{0}^\infty \frac{t^{-s}}{\sinh{(\pi t)}} \, dt.

Read more about this topic:  Dirichlet Eta Function

Famous quotes containing the word integral:

    “Painting myself for others, I have painted my inward self with colors clearer than my original ones. I have no more made my book than my book has made me—a book consubstantial with its author, concerned with my own self, an integral part of my life; not concerned with some third-hand, extraneous purpose, like all other books.”
    —Michel de Montaigne (1533–1592)