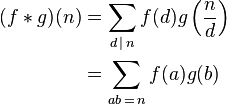Definition
If ƒ and g are two arithmetic functions (i.e. functions from the positive integers to the complex numbers), one defines a new arithmetic function ƒ * g, the Dirichlet convolution of ƒ and g, by
where the sum extends over all positive divisors d of n, or equivalently over all pairs (a, b) of positive integers whose product is n.
Read more about this topic: Dirichlet Convolution
Famous quotes containing the word definition:
“I’m beginning to think that the proper definition of “Man” is “an animal that writes letters.””
—Lewis Carroll [Charles Lutwidge Dodgson] (1832–1898)
“The very definition of the real becomes: that of which it is possible to give an equivalent reproduction.... The real is not only what can be reproduced, but that which is always already reproduced. The hyperreal.”
—Jean Baudrillard (b. 1929)
“The physicians say, they are not materialists; but they are:MSpirit is matter reduced to an extreme thinness: O so thin!—But the definition of spiritual should be, that which is its own evidence. What notions do they attach to love! what to religion! One would not willingly pronounce these words in their hearing, and give them the occasion to profane them.”
—Ralph Waldo Emerson (1803–1882)