Arithmetic Function

Arithmetic Function

In number theory, an arithmetic, arithmetical, or number-theoretic function is a real or complex valued function ƒ(n) defined on the set of natural numbers (i.e. positive integers) that "expresses some arithmetical property of n."

An example of an arithmetic function is the non-principal character (mod 4) defined by

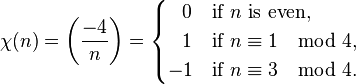\chi(n) = \left(\frac{-4}{n}\right)= \begin{cases} \;\;\,0 & \mbox{if } n \mbox{ is even}, \\ \;\;\, 1 & \mbox{if } n \equiv 1 \mod 4, \\ -1 & \mbox{if } n \equiv 3 \mod 4. \end{cases} where is the Kronecker symbol.

To emphasize that they are being thought of as functions rather than sequences, values of an arithmetic function are usually denoted by a(n) rather than an.

There is a larger class of number-theoretic functions that do not fit the above definition, e.g. the prime-counting functions. This article provides links to functions of both classes.

Read more about Arithmetic Function:  Notation, Multiplicative and Additive Functions, Ω(n), ω(n), νp(n) – Prime Power Decomposition, Summation Functions, Dirichlet Convolution, Relations Among The Functions

Famous quotes containing the words arithmetic and/or function:

    “‘Tis no extravagant arithmetic to say, that for every ten jokes,—thou hast got an hundred enemies; and till thou hast gone on, and raised a swarm of wasps about thine ears, and art half stung to death by them, thou wilt never be convinced it is so.”
    —Laurence Sterne (1713–1768)

    “The uses of travel are occasional, and short; but the best fruit it finds, when it finds it, is conversation; and this is a main function of life.”
    —Ralph Waldo Emerson (1803–1882)