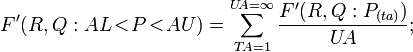Difference Quotient - Applying The Divided Difference

Applying The Divided Difference

The quintessential application of the divided difference is in the presentation of the definite integral, which is nothing more than a finite difference:

Given that the mean value, derivative expression form provides all of the same information as the classical integral notation, the mean value form may be the preferable expression, such as in writing venues that only support/accept standard ASCII text, or in cases that only require the average derivative (such as when finding the average radius in an elliptic integral). This is especially true for definite integrals that technically have (e.g.) 0 and either or as boundaries, with the same divided difference found as that with boundaries of 0 and (thus requiring less averaging effort):

This also becomes particularly useful when dealing with iterated and multiple integrals (ΔA = AU - AL, ΔB = BU - BL, ΔC = CU - CL):

=\sum_{T\!C=1}^{U\!C=\infty}\left(\sum_{T\!B=1}^{U\!B=\infty} \left(\sum_{T\!A=1}^{U\!A=\infty}F^'(R_{(tc)}:Q_{(tb)}:P_{(ta)})\frac{\Delta A}{U\!A}\right)\frac{\Delta B}{U\!B}\right)\frac{\Delta C}{U\!C},\,\!
= F'(C\!L\!<\!R\!<\!CU:BL\!<\!Q\!<\!BU:AL\!<\!P\!<\!AU) \Delta A\,\Delta B\,\Delta C .\,\!

Hence,

F'(R,Q:AL\!<\!P\!<\!AU)=\sum_{T\!A=1}^{U\!A=\infty} \frac{F'(R,Q:P_{(ta)})}{U\!A};\,\!

and

Read more about this topic:  Difference Quotient

Famous quotes containing the words applying the, applying, divided and/or difference:

    “There are always those who are willing to surrender local self-government and turn over their affairs to some national authority in exchange for a payment of money out of the Federal Treasury. Whenever they find some abuse needs correction in their neighborhood, instead of applying the remedy themselves they seek to have a tribunal sent on from Washington to discharge their duties for them, regardless of the fact that in accepting such supervision they are bartering away their freedom.”
    —Calvin Coolidge (1872–1933)

    “My old Father used to have a saying that “If you make a bad bargain, hug it the tighter”; and it occurs to me, that if the bargain you have just closed [marriage] can possibly be called a bad one, it is certainly the most pleasant one for applying that maxim to, which my fancy can, by any effort, picture.”
    —Abraham Lincoln (1809–1865)

    “O born in days when wits were fresh and clear,
    And life ran gaily as the sparkling Thames;
    Before this strange disease of modern life,
    With its sick hurry, its divided aims,
    Its head o’ertaxed, its palsied hearts, was rife—”
    —Matthew Arnold (1822–1888)

    “There is a difference between twenty-nine and thirty. When you are twenty-nine it can be the beginning of everything. When you are thirty it can be the end of everything.”
    —Gertrude Stein (1874–1946)