Decimal Representation - Finite Decimal Representations

Finite Decimal Representations

The decimal expansion of non-negative real number x will end in zeros (or in nines) if, and only if, x is a rational number whose denominator is of the form 2n5m, where m and n are non-negative integers.

Proof:

If the decimal expansion of x will end in zeros, or for some n, then the denominator of x is of the form 10n = 2n5n.

Conversely, if the denominator of x is of the form 2n5m, 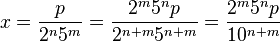x=\frac{p}{2^n5^m}=\frac{2^m5^np}{2^{n+m}5^{n+m}}= \frac{2^m5^np}{10^{n+m}} for some p. While x is of the form, for some n. By, x will end in zeros.

Read more about this topic:  Decimal Representation

Famous quotes containing the words finite and/or decimal:

    “We know then the existence and nature of the finite, because we also are finite and have extension. We know the existence of the infinite and are ignorant of its nature, because it has extension like us, but not limits like us. But we know neither the existence nor the nature of God, because he has neither extension nor limits.”
    —Blaise Pascal (1623–1662)

    “It makes little sense to spend a month teaching decimal fractions to fourth-grade pupils when they can be taught in a week, and better understood and retained, by sixth-grade students. Child-centeredness does not mean lack of rigor or standards; it does mean finding the best match between curricula and children’s developing interests and abilities.”
    —David Elkind (20th century)