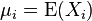Definition
Throughout this article, boldfaced unsubscripted X and Y are used to refer to random vectors, and unboldfaced subscripted Xi and Yi are used to refer to random scalars.
If the entries in the column vector
are random variables, each with finite variance, then the covariance matrix Σ is the matrix whose (i, j) entry is the covariance
where
is the expected value of the ith entry in the vector X. In other words, we have
The inverse of this matrix, is the inverse covariance matrix, also known as the concentration matrix or precision matrix; see precision (statistics). The elements of the precision matrix have an interpretation in terms of partial correlations and partial variances.
Read more about this topic: Covariance Matrix
Famous quotes containing the word definition:
“Mothers often are too easily intimidated by their children’s negative reactions...When the child cries or is unhappy, the mother reads this as meaning that she is a failure. This is why it is so important for a mother to know...that the process of growing up involves by definition things that her child is not going to like. Her job is not to create a bed of roses, but to help him learn how to pick his way through the thorns.”
—Elaine Heffner (20th century)
“Perhaps the best definition of progress would be the continuing efforts of men and women to narrow the gap between the convenience of the powers that be and the unwritten charter.”
—Nadine Gordimer (b. 1923)
“The very definition of the real becomes: that of which it is possible to give an equivalent reproduction.... The real is not only what can be reproduced, but that which is always already reproduced. The hyperreal.”
—Jean Baudrillard (b. 1929)