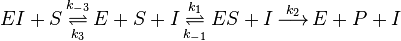Competitive Inhibition

Derivation

In the simplest case of a single-substrate enzyme obeying Michaelis-Menten kinetics, the typical scheme

$E + S \, \overset{k_1}\underset{k_{-1}} \rightleftharpoons \, ES \, \overset{k_2} {\longrightarrow} \, E + P$

is modified to include binding of the inhibitor to the free enzyme:

$EI + S \, \overset{k_{-3}}\underset{k_3} \rightleftharpoons \, E + S + I \, \overset{k_1}\underset{k_{-1}} \rightleftharpoons \, ES + I \, \overset{k_2} {\longrightarrow} \, E + P + I$

Note that the inhibitor does not bind to the ES complex and the substrate does not bind to the EI complex. It is generally assumed that this behavior is indicative of both compounds binding at the same site, but that is not strictly necessary. As with the derivation of the Michaelis-Menten equation, assume that the system is at steady-state, i.e. the concentration of each of the enzyme species is not changing.

Furthermore, the known total enzyme concentration is, and the velocity is measured under conditions in which the substrate and inhibitor concentrations do not change substantially and an insignificant amount of product has accumulated.

We can therefore set up a system of equations:

(1)

(2)

(3)

(4)

where, and are known. The initial velocity is defined as, so we need to define the unknown in terms of the knowns, and .

From equation (3), we can define E in terms of ES by rearranging to

Dividing by gives

As in the derivation of the Michaelis-Menten equation, the term can be replaced by the macroscopic rate constant :

(5)

Substituting equation (5) into equation (4), we have

Rearranging, we find that

At this point, we can define the dissociation constant for the inhibitor as, giving

(6)

At this point, substitute equation (5) and equation (6) into equation (1):

Rearranging to solve for ES, we find

(7)

Returning to our expression for, we now have:

$\begin{align} V_0 & = k_2 = \frac{k_2 K_i _0}{K_m K_i + K_i + K_m} \\ & = \frac{k_2 _0 }{K_m + + K_m\frac{}{K_i}} \end{align}$

Since the velocity is maximal when all the enzyme is bound as the enzyme-substrate complex, . Replacing and combining terms finally yields the conventional form: