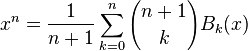Bernoulli Polynomials - Inversion

Inversion

The Bernoulli and Euler polynomials may be inverted to express the monomial in terms of the polynomials.

Specifically, evidently from the above section on #Representation by an integral operator, it follows that

x^n = \frac {1}{n+1} \sum_{k=0}^n {n+1 \choose k} B_k (x)

and

x^n = E_n (x) + \frac {1}{2} \sum_{k=0}^{n-1} {n \choose k} E_k (x).

Read more about this topic:  Bernoulli Polynomials