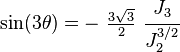Yield Surface - Invariants Used To Describe Yield Surfaces

Invariants Used To Describe Yield Surfaces

The first principal invariant of the Cauchy stress, and the second and third principal invariants of the deviatoric part of the Cauchy stress are defined as:

\begin{align} I_1 & = \text{Tr}(\boldsymbol{\sigma}) = \sigma_1 + \sigma_2 + \sigma_3 \\ J_2 & = \tfrac{1}{2} \boldsymbol{s}:\boldsymbol{s} = \tfrac{1}{6}\left \\ J_3 & = \det(\boldsymbol{s}) = \tfrac{1}{3} (\boldsymbol{s}\cdot\boldsymbol{s}):\boldsymbol{s} = s_1 s_2 s_3 \end{align}

where are the principal values of are the principal values of, and

\boldsymbol{s} = \boldsymbol{\sigma}-\tfrac{I_1}{3}\,\boldsymbol{I}

where is the identity matrix.

A related set of quantities, are usually used to describe yield surfaces for cohesive frictional materials such as rocks, soils, and ceramics. These are defined as

p = \tfrac{1}{3}~I_1 ~:~~ q = \sqrt{3~J_2} = \sigma_\mathrm{eq} ~;~~ r = 3\left(\tfrac{1}{2}\,J_3\right)^{1/3}

where is the equivalent stress. However, the possibility of negative values of and the resulting imaginary makes the use of these quantities problematic in practice.

Another related set of widely used invariants is which describe a cylindrical coordinate system (the Haigh–Westergaard coordinates). These are defined as:

\xi = \tfrac{1}{\sqrt{3}}~I_1 = \sqrt{3}~p ~;~~ \rho = \sqrt{2 J_2} = \sqrt{\tfrac{2}{3}}~q ~;~~ \cos(3\theta) = \left(\tfrac{r}{q}\right)^3 = \tfrac{3\sqrt{3}}{2}~\cfrac{J_3}{J_2^{3/2}}

The plane is also called the Rendulic plane. The angle is called the Lode angle and the relation between and was first given by Nayak and Zienkiewicz in 1972

The principal stresses and the Haigh–Westergaard coordinates are related by

\begin{bmatrix} \sigma_1 \\ \sigma_2 \\ \sigma_3 \end{bmatrix} = \tfrac{1}{\sqrt{3}} \begin{bmatrix} \xi \\ \xi \\ \xi \end{bmatrix} + \sqrt{\tfrac{2}{3}}~\rho~\begin{bmatrix} \cos\theta \\ \cos\left(\theta-\tfrac{2\pi}{3}\right) \\ \cos\left(\theta+\tfrac{2\pi}{3}\right) \end{bmatrix} = \tfrac{1}{\sqrt{3}} \begin{bmatrix} \xi \\ \xi \\ \xi \end{bmatrix} + \sqrt{\tfrac{2}{3}}~\rho~\begin{bmatrix} \cos\theta \\ -\sin\left(\tfrac{\pi}{6}-\theta\right) \\ -\sin\left(\tfrac{\pi}{6}+\theta\right) \end{bmatrix} \,.

A different definition of the Lode angle can also be found in the literature:

\sin(3\theta) = -~\tfrac{3\sqrt{3}}{2}~\cfrac{J_3}{J_2^{3/2}}

in which case

\begin{bmatrix} \sigma_1 \\ \sigma_2 \\ \sigma_3 \end{bmatrix} = \tfrac{1}{\sqrt{3}} \begin{bmatrix} \xi \\ \xi \\ \xi \end{bmatrix} + \sqrt{\tfrac{2}{3}}~\rho~\begin{bmatrix} \sin\left(\theta-\tfrac{2\pi}{3}\right) \\ \sin\theta \\ \sin\left(\theta+\tfrac{2\pi}{3}\right) \end{bmatrix} = \tfrac{1}{\sqrt{3}} \begin{bmatrix} \xi \\ \xi \\ \xi \end{bmatrix} + \sqrt{\tfrac{2}{3}}~\rho~\begin{bmatrix} -\cos\left(\tfrac{\pi}{6}-\theta\right) \\ \sin\theta \\ \cos\left(\tfrac{\pi}{6}+\theta\right) \end{bmatrix} \,.

Whatever definition is chosen, the angle varies between 0 degrees to +60 degrees.

Read more about this topic:  Yield Surface

Famous quotes containing the words describe, yield and/or surfaces:

    “As Cuvier could correctly describe a whole animal by the contemplation of a single bone, so the observer who has thoroughly understood one link in a series of incidents should be able to accurately state all the other ones, both before and after.”
    —Sir Arthur Conan Doyle (1859–1930)

    “Never yield to that temptation, which, to most young men, is very strong, of exposing other people’s weaknesses and infirmities, for the sake either of diverting the company, or of showing your own superiority. You may get the laugh on your side by it for the present; but you will make enemies by it for ever; and even those who laugh with you then, will, upon reflection, fear, and consequently hate you.”
    —Philip Dormer Stanhope, 4th Earl Chesterfield (1694–1773)

    “Footnotes are the finer-suckered surfaces that allow tentacular paragraphs to hold fast to the wider reality of the library.”
    —Nicholson Baker (b. 1957)