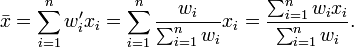Weighted Mean - Mathematical Definition

Mathematical Definition

Formally, the weighted mean of a non-empty set of data

with non-negative weights

is the quantity

\bar{x} = \frac{ \sum_{i=1}^n w_i x_i}{\sum_{i=1}^n w_i},

which means:

\bar{x} = \frac{w_1 x_1 + w_2 x_2 + \cdots + w_n x_n}{w_1 + w_2 + \cdots + w_n}.

Therefore data elements with a high weight contribute more to the weighted mean than do elements with a low weight. The weights cannot be negative. Some may be zero, but not all of them (since division by zero is not allowed).

The formulas are simplified when the weights are normalized such that they sum up to, i.e. . For such normalized weights the weighted mean is simply .

Note that one can always normalize the weights by making the following transformation on the weights . Using the normalized weight yields the same results as when using the original weights. Indeed,

\bar{x} = \sum_{i=1}^n w'_i x_i= \sum_{i=1}^n \frac{w_i}{\sum_{i=1}^n w_i} x_i = \frac{ \sum_{i=1}^n w_i x_i}{\sum_{i=1}^n w_i}.

The common mean is a special case of the weighted mean where all data have equal weights, . When the weights are normalized then

Read more about this topic:  Weighted Mean

Famous quotes containing the words mathematical and/or definition:

    “As we speak of poetical beauty, so ought we to speak of mathematical beauty and medical beauty. But we do not do so; and that reason is that we know well what is the object of mathematics, and that it consists in proofs, and what is the object of medicine, and that it consists in healing. But we do not know in what grace consists, which is the object of poetry.”
    —Blaise Pascal (1623–1662)

    “Mothers often are too easily intimidated by their children’s negative reactions...When the child cries or is unhappy, the mother reads this as meaning that she is a failure. This is why it is so important for a mother to know...that the process of growing up involves by definition things that her child is not going to like. Her job is not to create a bed of roses, but to help him learn how to pick his way through the thorns.”
    —Elaine Heffner (20th century)