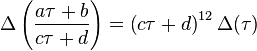Weierstrass's Elliptic Functions - Modular Discriminant

The modular discriminant Δ is defined as

This is studied in its own right, as a cusp form, in modular form theory (that is, as a function of the period lattice).

Note that where is the Dedekind eta function.

The presence of 24 can be understood by connection with other occurrences, as in the eta function and the Leech lattice.

The discriminant is a modular form of weight 12. That is, under the action of the modular group, it transforms as

$\Delta \left( \frac {a\tau+b} {c\tau+d}\right) = \left(c\tau+d\right)^{12} \Delta(\tau)$

with τ being the half-period ratio, and a,b,c and d being integers, with ad − bc = 1.