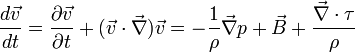Vorticity Equation - Derivation

Derivation

The vorticity equation can be derived from the Navier-Stokes equation for the conservation of angular momentum. In the absence of any concentrated torques and line forces, one obtains

$\frac{d \vec v}{d t} = \frac{\partial \vec v}{\partial t} + (\vec v \cdot \vec \nabla) \vec v = - \frac{1}{\rho} \vec \nabla p + \vec B + \frac{\vec \nabla \cdot \tau}{\rho}$

Now, vorticity is defined as the curl of the velocity vector. Taking curl of momentum equation yields the desired equation.

The following identities are useful in derivation of the equation,

, where is any scalar field.

Read more about this topic: Vorticity Equation