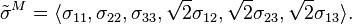Voigt Notation - Mandel Notation

Mandel Notation

For a symmetric tensor of second rank

\boldsymbol{\sigma}= \left[{\begin{matrix} \sigma_{11} & \sigma_{12} & \sigma_{13} \\ \sigma_{21} & \sigma_{22} & \sigma_{23} \\ \sigma_{31} & \sigma_{32} & \sigma_{33} \end{matrix}}\right]

only six components are distinct, the three on the diagonal and the other being off-diagonal. Thus it can be expressed, in Mandel notation, as the vector

\tilde \sigma ^M= \langle \sigma_{11}, \sigma_{22}, \sigma_{33}, \sqrt 2 \sigma_{12}, \sqrt 2 \sigma_{23}, \sqrt 2 \sigma_{13} \rangle.

The main advantage of Mandel notation is to allow the use of the same conventional operations used with vectors, for example:

\tilde \sigma : \tilde \sigma = \tilde \sigma^M \cdot \tilde \sigma^M = \sigma_{11}^2 + \sigma_{22}^2 + \sigma_{33}^2 + 2 \sigma_{12}^2 + 2 \sigma_{23}^2 + 2 \sigma_{13}^2.

A symmetric tensor of rank four satisfying and has 81 components in four-dimensional space, but only 36 components are distinct. Thus, in Mandel notation, it can be expressed as

\tilde D^M= \begin{pmatrix} D_{1111} & D_{1122} & D_{1133} & \sqrt 2 D_{1112} & \sqrt 2 D_{1123} & \sqrt 2 D_{1113} \\ D_{2211} & D_{2222} & D_{2233} & \sqrt 2 D_{2212} & \sqrt 2 D_{2223} & \sqrt 2 D_{2213} \\ D_{3311} & D_{3322} & D_{3333} & \sqrt 2 D_{3312} & \sqrt 2 D_{3323} & \sqrt 2 D_{3313} \\ \sqrt 2 D_{1211} & \sqrt 2 D_{1222} & \sqrt 2 D_{1233} & 2 D_{1212} & 2 D_{1223} & 2 D_{1213} \\ \sqrt 2 D_{2311} & \sqrt 2 D_{2322} & \sqrt 2 D_{2333} & 2 D_{2312} & 2 D_{2323} & 2 D_{2313} \\ \sqrt 2 D_{1311} & \sqrt 2 D_{1322} & \sqrt 2 D_{1333} & 2 D_{1312} & 2 D_{1323} & 2 D_{1313} \\ \end{pmatrix}.

Read more about this topic:  Voigt Notation