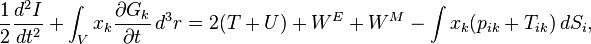Virial Theorem - Inclusion of Electromagnetic Fields

Inclusion of Electromagnetic Fields

The virial theorem can be extended to include electric and magnetic fields. The result is

\frac{1}{2}\frac{d^2I}{dt^2} + \int_Vx_k\frac{\partial G_k}{\partial t} \, d^3r = 2(T+U) + W^E + W^M - \int x_k(p_{ik}+T_{ik}) \, dS_i,

where I is the moment of inertia, G is the momentum density of the electromagnetic field, T is the kinetic energy of the "fluid", U is the random "thermal" energy of the particles, WE and WM are the electric and magnetic energy content of the volume considered. Finally, pik is the fluid-pressure tensor expressed in the local moving coordinate system

p_{ik} = \Sigma n^\sigma m^\sigma \langle v_iv_k\rangle^\sigma - V_iV_k\Sigma m^\sigma n^\sigma,

and Tik is the electromagnetic stress tensor,

T_{ik} = \left( \frac{\varepsilon_0E^2}{2} + \frac{B^2}{2\mu_0} \right) \delta_{ik} - \left( \varepsilon_0E_iE_k + \frac{B_iB_k}{\mu_0} \right).

A plasmoid is a finite configuration of magnetic fields and plasma. With the virial theorem it is easy to see that any such configuration will expand if not contained by external forces. In a finite configuration without pressure-bearing walls or magnetic coils, the surface integral will vanish. Since all the other terms on the right hand side are positive, the acceleration of the moment of inertia will also be positive. It is also easy to estimate the expansion time τ. If a total mass M is confined within a radius R, then the moment of inertia is roughly MR2, and the left hand side of the virial theorem is MR2/τ2. The terms on the right hand side add up to about pR3, where p is the larger of the plasma pressure or the magnetic pressure. Equating these two terms and solving for τ, we find

where cs is the speed of the ion acoustic wave (or the Alfvén wave, if the magnetic pressure is higher than the plasma pressure). Thus the lifetime of a plasmoid is expected to be on the order of the acoustic (or Alfvén) transit time.

Read more about this topic:  Virial Theorem

Famous quotes containing the words inclusion and/or fields:

    “Belonging to a group can provide the child with a variety of resources that an individual friendship often cannot—a sense of collective participation, experience with organizational roles, and group support in the enterprise of growing up. Groups also pose for the child some of the most acute problems of social life—of inclusion and exclusion, conformity and independence.”
    —Zick Rubin (20th century)

    “When we walk, we naturally go to the fields and woods: what would become of us, if we walked only in a garden or a mall?”
    —Henry David Thoreau (1817–1862)