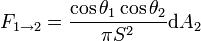View Factor - View Factors of Differential Areas

View Factors of Differential Areas

Taking the limit of a small flat surface gives differential areas, the view factor of two differential areas of areas and at a distance S is given by:

F_{1 \rarr 2} = \frac{\cos\theta_1 \cos\theta_2}{\pi S^2}\hbox{d}A_2

where and are the angle between the surface normals and a ray between the two differential areas.

The view factor from a general surface to another general surface is given by:

F_{1 \rarr 2} = \frac{1}{A_1} \int_{A_1} \int_{A_2} \frac{\cos\theta_1 \cos\theta_2}{\pi S^2}\, \hbox{d}A_2\, \hbox{d}A_1


The view factor is related to the etendue.

Read more about this topic:  View Factor

Famous quotes containing the words view, factors, differential and/or areas:

    “A man assumes that a woman’s refusal is just part of a game. Or, at any rate, a lot of men assume that. When a man says no, it’s no. When a woman says no, it’s yes, or at least maybe. There is even a joke to that effect. And little by little, women begin to believe in this view of themselves.”
    —Erica Jong (b. 1942)

    “The goal of every culture is to decay through over-civilization; the factors of decadence,—luxury, scepticism, weariness and superstition,—are constant. The civilization of one epoch becomes the manure of the next.”
    —Cyril Connolly (1903–1974)

    “But how is one to make a scientist understand that there is something unalterably deranged about differential calculus, quantum theory, or the obscene and so inanely liturgical ordeals of the precession of the equinoxes.”
    —Antonin Artaud (1896–1948)

    “... two great areas of deafness existed in the South: White Southerners had no ears to hear that which threatened their Dream. And colored Southerners had none to hear that which could reduce their anger.”
    —Sarah Patton Boyle, U.S. civil rights activist and author. The Desegregated Heart, part 1, ch. 16 (1962)