Van Der Waals Force - Van Der Waals Forces Between Macroscopic Objects

Van Der Waals Forces Between Macroscopic Objects

For macroscopic bodies with known volumes and numbers of atoms or molecules per unit volume, the total van der Waals force is often computed based on the "microscopic theory" as the sum over all interacting pairs. It is necessary to integrate over the total volume of the object, which makes the calculation dependent on the objects' shapes. For example, the van der Waals interaction energy between spherical bodies of radii R₁ and R₂ and with smooth surfaces was approximated in 1937 by Hamaker (using London's famous 1937 equation for the dispersion interaction energy between atoms/molecules as the starting point) by:

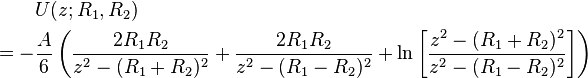
(1)

where A is the Hamaker coefficient, which is a constant (~10−19 − 10−20 J) that depends on the material properties (it can be positive or negative in sign depending on the intervening medium), and z is the center-to-center distance; i.e., the sum of R₁, R₂, and r (the distance between the surfaces): .

In the limit of close-approach, the spheres are sufficiently large compared to the distance between them; i.e., so that equation (1) for the potential energy function simplifies to:

(2)

The van der Waals force between two spheres of constant radii (R₁ and R₂ are treated as parameters) is then a function of separation since the force on an object is the negative of the derivative of the potential energy function,. This yields:

(3)

The van der Waals forces between objects with other geometries using the Hamaker model have been published in the literature.

From the expression above, it is seen that the van der Waals force decreases with decreasing particle size (R). Nevertheless, the strength of inertial forces, such as gravity and drag/lift, decrease to a greater extent. Consequently, the van der Waals forces become dominant for collections of very small particles such as very fine-grained dry powders (where there are no capillary forces present) even though the force of attraction is smaller in magnitude than it is for larger particles of the same substance. Such powders are said to be cohesive, meaning they are not as easily fluidized or pneumatically conveyed as easily as their more coarse-grained counterparts. Generally, free-flow occurs with particles greater than about 250 μm.

The van der Waals force of adhesion is also dependent on the surface topography. If there are surface asperities, or protuberances, that result in a greater total area of contact between two particles or between a particle and a wall, this increases the van der Waals force of attraction as well as the tendency for mechanical interlocking.

The microscopic theory assumes pairwise additivity. It neglects many-body interactions and retardation. A more rigorous approach accounting for these effects, called the "macroscopic theory," was developed by Lifshitz in 1956. Langbein derived a much more cumbersome "exact" expression in 1970 for spherical bodies within the framework of the Lifshitz theory while a simpler macroscopic model approximation had been made by Derjaguin as early as 1934. Expressions for the van der Waals forces for many different geometries using the Lifshitz theory have likewise been published.