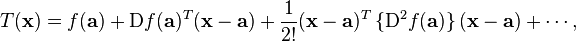Taylor Series - Taylor Series in Several Variables

Taylor Series in Several Variables

The Taylor series may also be generalized to functions of more than one variable with

T(x_1,\dots,x_d) = \sum_{n_1=0}^\infty \sum_{n_2=0}^\infty \cdots \sum_{n_d = 0}^\infty \frac{(x_1-a_1)^{n_1}\cdots (x_d-a_d)^{n_d}}{n_1!\cdots n_d!}\,\left(\frac{\partial^{n_1 + \cdots + n_d}f}{\partial x_1^{n_1}\cdots \partial x_d^{n_d}}\right)(a_1,\dots,a_d).\!

For example, for a function that depends on two variables, x and y, the Taylor series to second order about the point (a, b) is:

\begin{align} f(x,y) & \approx f(a,b) +(x-a)\, f_x(a,b) +(y-b)\, f_y(a,b) \\ & {}\quad + \frac{1}{2!}\left, \end{align}

where the subscripts denote the respective partial derivatives.

A second-order Taylor series expansion of a scalar-valued function of more than one variable can be written compactly as

T(\mathbf{x}) = f(\mathbf{a}) + \mathrm{D} f(\mathbf{a})^T (\mathbf{x} - \mathbf{a}) + \frac{1}{2!} (\mathbf{x} - \mathbf{a})^T \,\{\mathrm{D}^2 f(\mathbf{a})\}\,(\mathbf{x} - \mathbf{a}) + \cdots\! \,,

where is the gradient of evaluated at and is the Hessian matrix. Applying the multi-index notation the Taylor series for several variables becomes

which is to be understood as a still more abbreviated multi-index version of the first equation of this paragraph, again in full analogy to the single variable case.

Read more about this topic:  Taylor Series

Famous quotes containing the words taylor, series and/or variables:

    “Who fed me from her gentle breast,
    And hushed me in her arms to rest,
    And on my cheek sweet kisses prest?
    My Mother.”
    —Ann Taylor (1783–1824)

    “I thought I never wanted to be a father. A child seemed to be a series of limitations and responsibilities that offered no reward. But when I experienced the perfection of fatherhood, the rest of the world remade itself before my eyes.”
    —Kent Nerburn (20th century)

    “Science is feasible when the variables are few and can be enumerated; when their combinations are distinct and clear. We are tending toward the condition of science and aspiring to do it. The artist works out his own formulas; the interest of science lies in the art of making science.”
    —Paul Valéry (1871–1945)