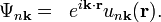Surface States - Origin of Surface States at Condensed Matter Interfaces

Origin of Surface States At Condensed Matter Interfaces

As stated by Bloch's theorem, eigenstates of the single-electron Schrödinger equation with a perfectly periodic potential, a crystal, are Bloch waves

\begin{align} \Psi_{n\textbf{k}} &=& e^{i\textbf{k}\cdot\textbf{r}}u_{n\textbf{k}}(\textbf{r}). \end{align}

Here is a function with the same periodicity as the crystal, n is the band index and k is the wave number. The allowed wave numbers for a given potential are found by applying the usual Born–von Karman cyclic boundary conditions . The termination of a crystal, i.e. the formation of a surface, obviously causes deviation from perfect periodicity. Consequently, if the cyclic boundary conditions are abandoned in the direction normal to the surface the behavior of electrons will deviate from the behavior in the bulk and some modifications of the electronic structure has to be expected.

A simplified model of the crystal potential in one dimension can be sketched as shown in figure 1 . In the crystal, the potential has the periodicity, a, of the lattice while close to the surface it has to somehow attain the value of the vacuum level. The step potential (solid line) shown in figure 1 is an oversimplification which is mostly convenient for simple model calculations. At a real surface the potential is influenced by image charges and the formation of surface dipoles and it rather looks as indicated by the dashed line.

Given the potential in figure 1, it can be shown that the one-dimensional single-electron Schrödinger equation gives two qualitatively different types of solutions.

  • The first type of states (see figure 2) extends into the crystal and has Bloch character there. These type of solutions correspond to bulk states which terminate in an exponentially decaying tail reaching into the vacuum.
  • The second type of states (see figure 3) decays exponentially both into the vacuum and the bulk crystal. These type of solutions correspond to states, with wave functions localized close to the crystal surface.

The first type of solution can be obtained for both metals and semiconductors. In semiconductors though, the associated eigenenergies have to belong to one of the allowed energy bands. The second type of solution exists in forbidden energy gap of semiconductors as well as in local gaps of the projected band structure of metals. It can be shown that the energies of these states all lie within the band gap. As a consequence, in the crystal these states are characterized by an imaginary wavenumber leading to an exponential decay into the bulk.

Read more about this topic:  Surface States

Famous quotes containing the words origin of, origin, surface, states, condensed and/or matter:

    “The real, then, is that which, sooner or later, information and reasoning would finally result in, and which is therefore independent of the vagaries of me and you. Thus, the very origin of the conception of reality shows that this conception essentially involves the notion of a COMMUNITY, without definite limits, and capable of a definite increase of knowledge.”
    —Charles Sanders Peirce (1839–1914)

    “Someone had literally run to earth
    In an old cellar hole in a byroad
    The origin of all the family there.
    Thence they were sprung, so numerous a tribe
    That now not all the houses left in town
    Made shift to shelter them without the help
    Of here and there a tent in grove and orchard.”
    —Robert Frost (1874–1963)

    “Night City was like a deranged experiment in Social Darwinism, designed by a bored researcher who kept one thumb permanently on the fast-forward button. Stop hustling and you sank without a trace, but move a little too swiftly and you’d break the fragile surface tension of the black market; either way, you were gone ... though heart or lungs or kidneys might survive in the service of some stranger with New Yen for the clinic tanks.”
    —William Gibson (b. 1948)

    “Money is power, and in that government which pays all the public officers of the states will all political power be substantially concentrated.”
    —Andrew Jackson (1767–1845)

    “There is no doubt that the loftiest written wisdom is either rhymed or in some way musically measured,—is, in form as well as substance, poetry; and a volume which should contain the condensed wisdom of mankind need not have one rhythmless line.”
    —Henry David Thoreau (1817–1862)

    “What does it matter if, by chance, a little vile blood be spilled?”
    —Jean Racine (1639–1699)