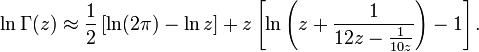Versions Suitable For Calculators
The approximation:
or equivalently,
can be obtained by rearranging Stirling's extended formula and observing a coincidence between the resultant power series and the Taylor series expansion of the hyperbolic sine function. This approximation is good to more than 8 decimal digits for z with a real part greater than 8. Robert H. Windschitl suggested it in 2002 for computing the Gamma function with fair accuracy on calculators with limited program or register memory.
Gergő Nemes proposed in 2007 an approximation which gives the same number of exact digits as the Windschitl approximation but is much simpler:
or equivalently,
An apparently superior approximation for log n! was also given by Srinivasa Ramanujan (Ramanujan 1988)
Read more about this topic: Stirling's Approximation
Famous quotes containing the words versions, suitable and/or calculators:
“The assumption must be that those who can see value only in tradition, or versions of it, deny man’s ability to adapt to changing circumstances.”
—Stephen Bayley (b. 1951)
“Not one of our mortal gauges is suitable for evaluating non-existence, for making judgments about that which is not a person.”
—Anton Pavlovich Chekhov (1860–1904)
“But the age of chivalry is gone. That of sophisters, economists, and calculators has succeeded; and the glory of Europe is extinguished forever.”
—Edmund Burke (1729–1797)