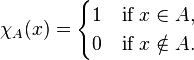Definition and First Consequences
A function is called a step function if it can be written as
- for all real numbers
where are real numbers, are intervals, and is the indicator function of :
In this definition, the intervals can be assumed to have the following two properties:
- The intervals are disjoint, for
- The union of the intervals is the entire real line,
Indeed, if that is not the case to start with, a different set of intervals can be picked for which these assumptions hold. For example, the step function
can be written as
Read more about this topic: Step Function
Famous quotes containing the words definition and/or consequences:
“Scientific method is the way to truth, but it affords, even in
principle, no unique definition of truth. Any so-called pragmatic
definition of truth is doomed to failure equally.”
—Willard Van Orman Quine (b. 1908)
“Without being forgiven, released from the consequences of what we have done, our capacity to act would ... be confined to one single deed from which we could never recover; we would remain the victims of its consequences forever, not unlike the sorcerer’s apprentice who lacked the magic formula to break the spell.”
—Hannah Arendt (1906–1975)