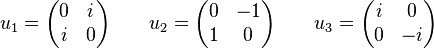Special Unitary Group - n = 2

n = 2

SU(2) is the following group:

where the overline denotes complex conjugation. Now consider the following map:

where M(2, C) denotes the set of 2 by 2 complex matrices. By considering C2 diffeomorphic to R4 and M(2, C) diffeomorphic to R8 we can see that φ is an injective real linear map and hence an embedding. Now considering the restriction of φ to the 3-sphere, denoted S3, we can see that this is an embedding of the 3-sphere onto a compact submanifold of M(2, C). However it is also clear that φ(S3) = SU(2). Therefore as a manifold S3 is diffeomorphic to SU(2) and so SU(2) is a compact, connected Lie group.

The Lie algebra of SU(2) is:

It is easily verified that matrices of this form have trace zero and are antihermitian. The Lie algebra is then generated by the following matrices

which are easily seen to have the form of the general element specified above. These satisfy u₃u₂ = −u₂u₃ = −u₁ and u₂u₁ = −u₁u₂ = −u₃. The commutator bracket is therefore specified by

The above generators are related to the Pauli matrices by u₁ = i σ₁,u₂ = −i σ₂ and u₃ = i σ₃. This representation is often used in quantum mechanics (see Pauli matrices and Gell-Mann matrices), to represent the spin of fundamental particles such as electrons. They also serve as unit vectors for the description of our 3 spatial dimensions in loop quantum gravity.