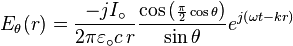Signal Strength - Relationship To Average Radiated Power

Relationship To Average Radiated Power

The electric field strength at a specific point can be determined from the power delivered to the transmitting antenna, its geometry and radiation resistance. Consider the case of a center-fed half-wave dipole antenna in free space . If constructed from thin conductors, the current distribution is essentially sinusoidal and the radiating electric field is given by

E_\theta (r) = {-jI_\circ\over 2\pi\varepsilon_\circ c\, r} {\cos\left(\scriptstyle{\pi\over 2}\cos\theta\right)\over\sin\theta} e^{j\left(\omega t-kr\right)}

where is the angle between the antenna axis and the vector to the observation point, is the peak current at the feed-point, is the permittivity of free-space, is the speed of light in a vacuum, and is the distance to the antenna in meters. When the antenna is viewed broadside the electric field is maximum and given by

\vert E_{\pi/2}(r) \vert = { I_\circ \over 2\pi\varepsilon_\circ c\, r }\, .

Solving this formula for the peak current yields

I_\circ = 2\pi\varepsilon_\circ c \, r\vert E_{\pi/2}(r) \vert \, .

The average power to the antenna is

where is the center-fed half-wave antenna’s radiation resistance. Substituting the formula for into the one for and solving for the maximum electric field yields

\vert E_{\pi/2}(r)\vert \, = \, {1 \over \pi\varepsilon_\circ c \, r} \sqrt{{ P_{avg} \over 2R_a}} \, = \, {9.91 \over r} \sqrt{ P_{avg} } \quad (L = \lambda /2) \, .

Therefore, if the average power to a half-wave dipole antenna is 1 mW, then the maximum electric field at 313 m (1027 ft) is 1 mV/m (60 dBµ).

For a short dipole the current distribution is nearly triangular. In this case, the electric field and radiation resistance are

E_\theta (r) = {-jI_\circ \sin (\theta) \over 4 \varepsilon_\circ c\, r} \left ( {L \over \lambda} \right ) e^{j\left(\omega t-kr\right)} \, \quad R_a = 20\pi^2 \left ( {L \over \lambda} \right )^2 .

Using a procedure similar to that above, the maximum electric field for a center-fed short dipole is

\vert E_{\pi/2}(r)\vert \, = \, {1 \over \pi\varepsilon_\circ c \, r} \sqrt{{ P_{avg} \over 160}} \, = \, {9.48 \over r} \sqrt{ P_{avg} } \quad (L \ll \lambda /2)\, .

Read more about this topic:  Signal Strength

Famous quotes containing the words relationship to, relationship, average and/or power:

    “... the Wall became a magnet for citizens of every generation, class, race, and relationship to the war perhaps because it is the only great public monument that allows the anesthetized holes in the heart to fill with a truly national grief.”
    —Adrienne Rich (b. 1929)

    “When any relationship is characterized by difference, particularly a disparity in power, there remains a tendency to model it on the parent-child-relationship. Even protectiveness and benevolence toward the poor, toward minorities, and especially toward women have involved equating them with children.”
    —Mary Catherine Bateson (20th century)

    “...you don’t have to be as good as white people, you have to be better or the best. When Negroes are average, they fail, unless they are very, very lucky. Now, if you’re average and white, honey, you can go far. Just look at Dan Quayle. If that boy was colored he’d be washing dishes somewhere.”
    —Annie Elizabeth Delany (b. 1891)

    “No punishment has ever possessed enough power of deterrence to prevent the commission of crimes. On the contrary, whatever the punishment, once a specific crime has appeared for the first time, its reappearance is more likely than its initial emergence could ever have been.”
    —Hannah Arendt (1906–1975)