Rotation Matrix - in Two Dimensions

In Two Dimensions

In two dimensions every rotation matrix has the following form:

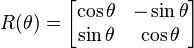R(\theta) = \begin{bmatrix} \cos \theta & -\sin \theta \\ \sin \theta & \cos \theta \\ \end{bmatrix}.

This rotates column vectors by means of the following matrix multiplication:

\begin{bmatrix} x' \\ y' \\ \end{bmatrix} = \begin{bmatrix} \cos \theta & -\sin \theta \\ \sin \theta & \cos \theta \\ \end{bmatrix}\begin{bmatrix} x \\ y \\ \end{bmatrix}.

So the coordinates (x',y') of the point (x,y) after rotation are:

,
.

The direction of vector rotation is counterclockwise if θ is positive (e.g. 90°), and clockwise if θ is negative (e.g. -90°).

R(-\theta) = \begin{bmatrix} \cos \theta & \sin \theta \\ -\sin \theta & \cos \theta \\ \end{bmatrix}\,.

Note that the two-dimensional case is the only non-trivial (e.g. one dimension) case where the rotation matrices group is commutative, so that it does not matter the order in which multiple rotations are performed.

Read more about this topic:  Rotation Matrix

Famous quotes containing the word dimensions:

    “It seems to me that we do not know nearly enough about ourselves; that we do not often enough wonder if our lives, or some events and times in our lives, may not be analogues or metaphors or echoes of evolvements and happenings going on in other people?—or animals?—even forests or oceans or rocks?—in this world of ours or, even, in worlds or dimensions elsewhere.”
    —Doris Lessing (b. 1919)