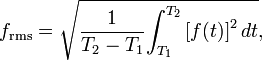Root Mean Square - Definition

Definition

The RMS value of a set of values (or a continuous-time waveform) is the square root of the arithmetic mean (average) of the squares of the original values (or the square of the function that defines the continuous waveform).

In the case of a set of values, the RMS value is given by this formula:

x_{\mathrm{rms}} = \sqrt{ \frac{1}{n} \left( x_1^2 + x_2^2 + \cdots + x_n^2 \right) }

The corresponding formula for a continuous function (or waveform) defined over the interval is

f_{\mathrm{rms}} = \sqrt {{1 \over {T_2-T_1}} {\int_{T_1}^{T_2} {}^2\, dt}},

and the RMS for a function over all time is

f_\mathrm{rms} = \lim_{T\rightarrow \infty} \sqrt {{1 \over {T}} {\int_{0}^{T} {}^2\, dt}}.

The RMS over all time of a periodic function is equal to the RMS of one period of the function. The RMS value of a continuous function or signal can be approximated by taking the RMS of a series of equally spaced samples. Additionally, the RMS value of various waveforms can also be determined without calculus, as shown by Cartwright.

In the case of the RMS statistic of a random process, the expected value is used instead of the mean.

Read more about this topic:  Root Mean Square

Famous quotes containing the word definition:

    “Although there is no universal agreement as to a definition of life, its biological manifestations are generally considered to be organization, metabolism, growth, irritability, adaptation, and reproduction.”
    —The Columbia Encyclopedia, Fifth Edition, the first sentence of the article on “life” (based on wording in the First Edition, 1935)

    “I’m beginning to think that the proper definition of “Man” is “an animal that writes letters.””
    —Lewis Carroll [Charles Lutwidge Dodgson] (1832–1898)

    “The physicians say, they are not materialists; but they are:MSpirit is matter reduced to an extreme thinness: O so thin!—But the definition of spiritual should be, that which is its own evidence. What notions do they attach to love! what to religion! One would not willingly pronounce these words in their hearing, and give them the occasion to profane them.”
    —Ralph Waldo Emerson (1803–1882)