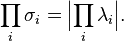Connection To A Determinant or A Product of Eigenvalues
We can use QR decomposition to find the absolute value of the determinant of a square matrix. Suppose a matrix is decomposed as . Then we have
Since Q is unitary, . Thus,
where are the entries on the diagonal of R.
Furthermore, because the determinant equals the product of the eigenvalues, we have
where are eigenvalues of .
We can extend the above properties to non-square complex matrix by introducing the definition of QR-decomposition for non-square complex matrix and replacing eigenvalues with singular values.
Suppose a QR decomposition for a non-square matrix A:
where is a zero matrix and is an unitary matrix.
From the properties of SVD and determinant of matrix, we have
where are singular values of .
Note that the singular values of and are identical, although the complex eigenvalues of them may be different. However, if A is square, it holds that
In conclusion, QR decomposition can be used efficiently to calculate a product of eigenvalues or singular values of matrix.
Read more about this topic: QR Decomposition
Famous quotes containing the words connection to, connection and/or product:
“One must always maintain one’s connection to the past and yet ceaselessly pull away from it. To remain in touch with the past requires a love of memory. To remain in touch with the past requires a constant imaginative effort.”
—Gaston Bachelard (1884–1962)
“We live in a world of things, and our only connection with them is that we know how to manipulate or to consume them.”
—Erich Fromm (1900–1980)
“Poetry is the only life got, the only work done, the only pure product and free labor of man, performed only when he has put all the world under his feet, and conquered the last of his foes.”
—Henry David Thoreau (1817–1862)