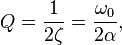Q Factor - Physical Interpretation of Q

Physical Interpretation of Q

Physically Speaking, Q is times the ratio of the total energy stored divided by the energy lost in a single cycle or equivalently the ratio of the stored energy to the energy dissipated over one radian of the oscillation.

It is a dimensionless parameter that compares the time constant for decay of an oscillating physical system's amplitude to its oscillation period. Equivalently, it compares the frequency at which a system oscillates to the rate at which it dissipates its energy.

Equivalently (for large values of Q), the Q factor is approximately the number of oscillations required for a freely oscillating system's energy to fall off to, or about 1/535 or 0.2%, of its original energy.

The width (bandwidth) of the resonance is given by

\Delta f = \frac{f_0}{Q} \, ,

where is the resonant frequency, and, the bandwidth, is the width of the range of frequencies for which the energy is at least half its peak value.

The factors Q, damping ratio ζ, and attenuation α are related such that

\zeta = \frac{1}{2 Q} = { \alpha \over \omega_0 }.

So the quality factor can be expressed as

Q = \frac{1}{2 \zeta} = { \omega_0 \over 2 \alpha },

and the exponential attenuation rate can be expressed as

\alpha = \zeta \omega_0 = { \omega_0 \over 2 Q }.

For any 2nd order low-pass filter, the response function of the filter is

H(s) = \frac{ \omega_0^2 }{ s^2 + \underbrace{ \frac{ \omega_0 }{Q} }_{2 \zeta \omega_0 = 2 \alpha }s + \omega_0^2 } \,

For this system, when (i.e., when the system is underdamped), it has two complex conjugate poles that each have a real part of . That is, the attenuation parameter represents the rate of exponential decay of the oscillations (e.g., after an impulse) of the system. A higher quality factor implies a lower attenuation, and so high Q systems oscillate for long times. For example, high quality bells have an approximately pure sinusoidal tone for a long time after being struck by a hammer.

Read more about this topic:  Q Factor

Famous quotes containing the word physical:

    “The real stumbling-block of totalitarian régimes is not the spiritual need of men for freedom of thought; it is men’s inability to stand the physical and nervous strain of a permanent state of excitement, except during a few years of their youth.”
    —Simone Weil (1909–1943)