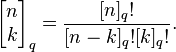Relationship To Other Q-functions
Noticing that
we define the q-analog of n, also known as the q-bracket or q-number of n to be
From this one can define the q-analog of the factorial, the q-factorial, as
Again, one recovers the usual factorial by taking the limit as q approaches 1. This can be interpreted as the number of flags in an n-dimensional vector space over the field with q elements, and taking the limit as q goes to 1 yields the interpretation of an ordering on a set as a flag in a vector space over the field with one element.
A product of negative integer q-brackets can be expressed in terms of the q-factorial as:
From the q-factorials, one can move on to define the q-binomial coefficients, also known as Gaussian coefficients, Gaussian polynomials, or Gaussian binomial coefficients:
One can check that
One also obtains a q-analog of the Gamma function, called the q-gamma function, and defined as
This converges to the usual Gamma function as q approaches 1 from inside the unit disc.. Note that
for any x and
for non-negative integer values of n. Alternatively, this may be taken as an extension of the q-factorial function to the real number system.
Read more about this topic: Q-Pochhammer Symbol
Famous quotes containing the words relationship to and/or relationship:
“... the Wall became a magnet for citizens of every generation, class, race, and relationship to the war perhaps because it is the only great public monument that allows the anesthetized holes in the heart to fill with a truly national grief.”
—Adrienne Rich (b. 1929)
“The relationship between mother and professional has not been a partnership in which both work together on behalf of the child, in which the expert helps the mother achieve her own goals for her child. Instead, professionals often behave as if they alone are advocates for the child; as if they are the guardians of the child’s needs; as if the mother left to her own devices will surely damage the child and only the professional can rescue him.”
—Elaine Heffner (20th century)