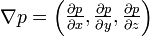Mathematical Description
Assuming that the pressure p is an intensive quantity, i.e., a single-valued, continuous and differentiable function of three-dimensional space (often called a scalar field), i.e., that
where x, y and z are the coordinates of the location of interest, then the pressure gradient is the vector quantity defined as
Read more about this topic: Pressure Gradient
Famous quotes containing the words mathematical and/or description:
“The most distinct and beautiful statement of any truth must take at last the mathematical form.”
—Henry David Thoreau (1817–1862)
“The next Augustan age will dawn on the other side of the Atlantic. There will, perhaps, be a Thucydides at Boston, a Xenophon at New York, and, in time, a Virgil at Mexico, and a Newton at Peru. At last, some curious traveller from Lima will visit England and give a description of the ruins of St Paul’s, like the editions of Balbec and Palmyra.”
—Horace Walpole (1717–1797)