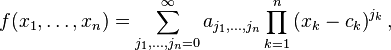Power Series - Power Series in Several Variables

Power Series in Several Variables

An extension of the theory is necessary for the purposes of multivariable calculus. A power series is here defined to be an infinite series of the form

f(x_1,\dots,x_n) = \sum_{j_1,\dots,j_n = 0}^{\infty}a_{j_1,\dots,j_n} \prod_{k=1}^n \left(x_k - c_k \right)^{j_k},

where j = (j1, ..., jn) is a vector of natural numbers, the coefficients a(j1,...,jn) are usually real or complex numbers, and the center c = (c1, ..., cn) and argument x = (x1, ..., xn) are usually real or complex vectors. In the more convenient multi-index notation this can be written

f(x) = \sum_{\alpha \in \mathbb{N}^n} a_{\alpha} \left(x - c \right)^{\alpha}.

The theory of such series is trickier than for single-variable series, with more complicated regions of convergence. For instance, the power series is absolutely convergent in the set between two hyperbolas. (This is an example of a log-convex set, in the sense that the set of points, where lies in the above region, is a convex set. More generally, one can show that when c=0, the interior of the region of absolute convergence is always a log-convex set in this sense.) On the other hand, in the interior of this region of convergence one may differentiate and integrate under the series sign, just as one may with ordinary power series.

Read more about this topic:  Power Series

Famous quotes containing the words power, series and/or variables:

    “An actor must communicate his author’s given message—comedy, tragedy, serio- comedy; then comes his unique moment, as he is confronted by the looked-for, yet at times unexpected, reaction of the audience. This split second is his; he is in command of his medium; the effect vanishes into thin air; but that moment has a power all its own and, like power in any form, is stimulating and alluring.”
    —Eleanor Robson Belmont (1878–1979)

    “Depression moods lead, almost invariably, to accidents. But, when they occur, our mood changes again, since the accident shows we can draw the world in our wake, and that we still retain some degree of power even when our spirits are low. A series of accidents creates a positively light-hearted state, out of consideration for this strange power.”
    —Jean Baudrillard (b. 1929)

    “Science is feasible when the variables are few and can be enumerated; when their combinations are distinct and clear. We are tending toward the condition of science and aspiring to do it. The artist works out his own formulas; the interest of science lies in the art of making science.”
    —Paul Valéry (1871–1945)